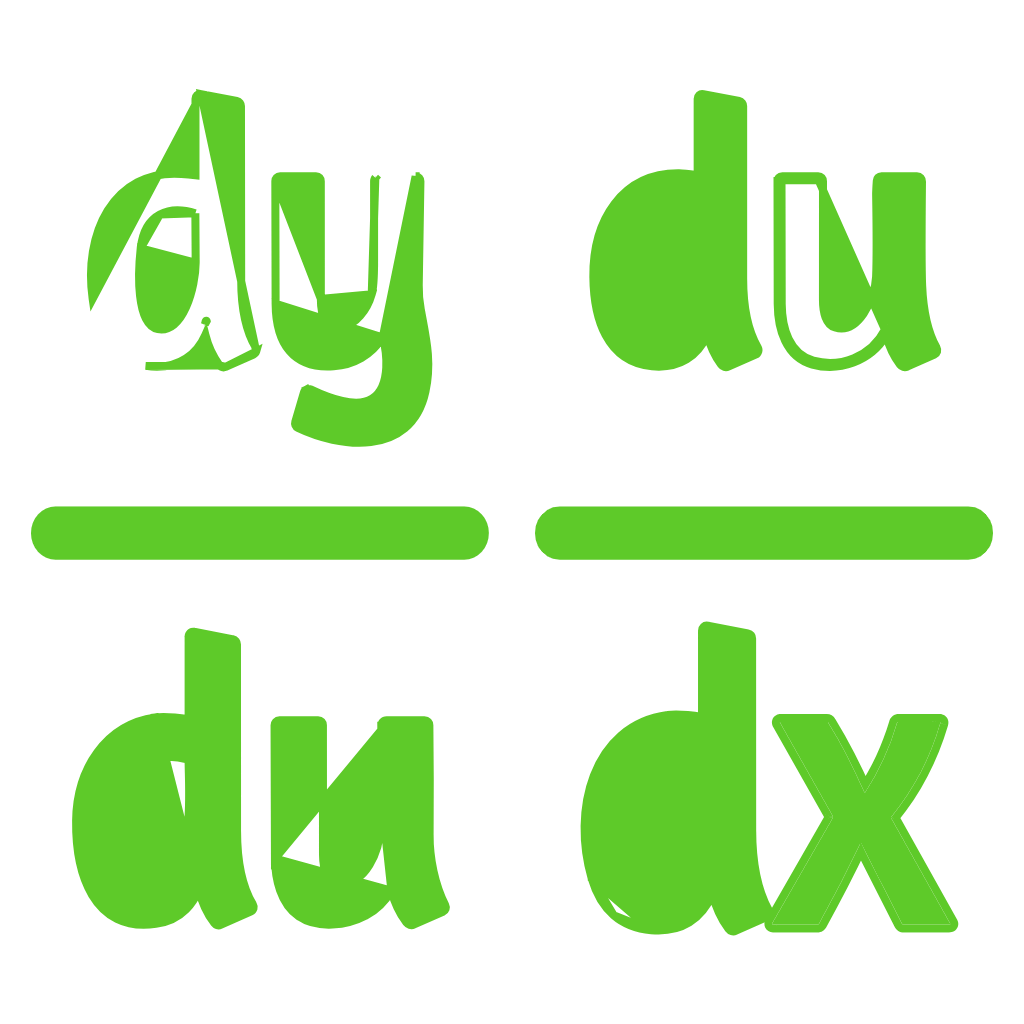Introduction to Derivative at a Point Calculator
The derivative calculator at a point is an online differential tool that helps you evaluate a function derivative at a given point. It allows the user to compute the average rate of change of the differential function at a particular point.
Our derivative at point calculator gives a graphical representation for visual understanding. Whether you're a student grappling with calculus concepts or a professional in need of quick and accurate derivative calculations, this calculator serves as your go-to solution.
By inputting the function and the desired point of evaluation, the calculator swiftly computes the derivative using rigorous mathematical algorithms.
While the Derivative at a Point Calculator focuses on practical computation of derivatives at specific points, the Calculator offers a deeper understanding of the theoretical basis of differentiation. you may use the definition of a derivative calculator to reinforce their understanding of the fundamental definition of the derivative and the concept of limits.
What is the Derivative at a Point?
The derivative of a function at a point is defined as the instantaneous rate of change of a function at a specific point. It gives the exact slope along with the curve at that point.
The derivative at a point provides valuable information about the instantaneous rate of change of the function at that specific location. It helps in understanding the behavior of functions, identifying critical points such as maximums, minimums, and inflection points, and analyzing real-world phenomena involving rates of change
While the Derivative at a Point Calculator provides a precise value of the derivative at a single point, the Slope of the Curve Calculator offers a broader perspective by visualizing the slope of the curve over an interval.
Users can use the Derivative at a Point Calculator to obtain specific derivative values at critical points, while the slope of a curve at a point calculator helps in understanding the overall trend and behavior of the function over a given range.
Formula behind Derivative Calculator at a Point
The derivative at a point formula is used to evaluate derivative at a point by replacing x with that specific point as ax. The formula used by our derivative at a point calculator is,
$$ f’(a) \;=\; \lim_{h \to 0} \frac{f(a+h) - f(a)}{h} $$
f`(a)= the derivative of f(a)
h= change in a.
f(a)=the original function.
f(a+h)=change in f(a) due to the change in a.
This formula encapsulates the concept of the derivative at a point, where we evaluate the rate of change of the function as the interval ℎ approaches zero. By substituting the specific point a into the formula and computing the limit as ℎ approaches zero, we obtain the derivative of the function at that particular point.
Additionally, for further analysis and visualization of derivatives, you can use our derivative graphing calculator. This calculator enables users to plot the graph of a function along with its derivative, providing a comprehensive visual representation for enhanced understanding and analysis.
Evaluation Process of Derivative at Point Calculator
Point differential calculator uses the fundamental differential formula so you can calculate the rate of change function at any specific point as all derivative rules are already built like product, quotient, and chain rule.
Your input function and the point depend on the working of this dy/dx at a point calculator. When you give a derivative at a point function as input, it substitutes the derivative limit formula f`(a) a point a to find the rate of change at a particular point.
Then, the calculation of f`(a), excludes h from the denominator because if the calculator applies limit h which is in the denominator tends to zero and makes the function undefined.
After reducing, the derivative at a point calculator allows limits to apply and give the result of the derivative at a point problem in the run of time.
While the Derivative at Point Calculator focuses on computing the rate of change of a function at a specific point, you can use our derivative of the inverse calculator that deals with finding the derivatives of inverse functions.
Our calculator also provides a convenient way to compute the derivative of inverse functions at specific points.
Now let's understand the working of this calculator with an example.
Example of the derivative at a point:
An example of derivative point function to understand the differential concept at a specific point a is given,
Example:
Find derivative at a point for the given function. Use the limit definition of the derivative to calculate f’(2):
$$ f(x) \;=\; x - x^2 $$
Solution:
Now use the rule for f and observe that f(2) = 2-22 and f(2+h) = (2+h) - (2+h)2.
$$ f’(2) \;=\; \lim_{h \to 0} \frac{(2+h) - (2+h)^2 - (-2)}{h} $$
$$ f’(2) \;=\; \lim_{h \to 0} {2 + h - 4 - 4h -h^2 + 2 \over h } $$
Combining the like terms,
$$ f’(2) \;=\; \lim_{h \to 0} \frac{-3h - h^2}{h} $$
Now simplify as the nominator and denominator have the common factor of h,
$$ f’(2) \;=\; \lim_{h \to 0} (-3 - h) $$
Thus we conclude that f’(2) = -3.
Thus it is the final solution of our function with specific limits. Additionally, for further analysis and computation of second implicit derivatives, you can utilize our d2y/dx2 calculator.
This tool provides a convenient way to compute the second derivative of implicitly defined functions, offering insights into the curvature and behavior of the curve.
How does Derivative at a Point Calculator work?
Our derivative calculator at a point will help you to calculate first or second-order derivation at a given point on the tangent line. To find the accurate value, you should follow these simple steps while using the dy/dx at a point calculator. These steps are:
Step 1:
Type the function in the given input field.
Step 2:
Select the order of derivative for your function
Step 3:
Add the specific point at which you differentiate your function
Step 4:
Click on the "calculate" button to get the desired derivative.
Additionally, if you would like to compute the derivative of an implicitly defined function with respect to a given variable, you can use our dy/dx calculator. Our calculator also helps in finding derivatives when the dependent variable cannot be explicitly expressed in terms of the independent variable.
Result Obtained from Point Differential Calculator
You will get the result after entering the input function in this derivative at a point calculator. It will show different points.
These points are:
- Solution along with the given input function
- Possible step solution section will give you throughout method of calculation in steps
- Plot section will provide a graph of your function
- The Recalculate button helps you to calculate more examples.
Advantages of Using a dy/dx at a Point Calculator
Derivative at a point calculator helps you to improve your problem-solving skills in less time and gives multiple benefits. These are:
- It helps you to find derivatives at a point by giving input values only.
- derivative at point calculator improves your analytical skills faster.
- It is a free tool and available online
- It calculates derivatives quickly and accurately as it provides steps.
- Our point differential calculator is very beneficial to those who do not have a good command of differentiation concepts.
- Our calculator serves as a valuable learning tool for individuals seeking to enhance their understanding of differentiation concepts. Its user-friendly interface and step-by-step solutions make it particularly beneficial for users who are less familiar with differentiation principles.
Further, for handling complex logarithmic functions and exploring logarithmic differentiation, you can go through our logarithmic derivative calculator. This calculator simplifies the process of finding derivatives of logarithmic functions, providing step-by-step solutions for enhanced understanding and learning.
Be Attentive while Using this Calculator
Users should check these errors which are mentioned below to get accurate or better results.
- Your expression of the function that you would like to differentiate should be placed in the input box with your particular point.
- Make sure that the function depends on the differentiation variable you specified.
- Make sure the expression you are differentiating is written in the correct structure so that there is no red error found on the derivative at a point calculator.
Additionally, for a comprehensive collection of mathematical tools and calculators, including the Implicit Differentiation Calculator, explore our All Calculators section. This repository offers a wide array of calculators to cater to diverse mathematical needs and assist you in various computational tasks.