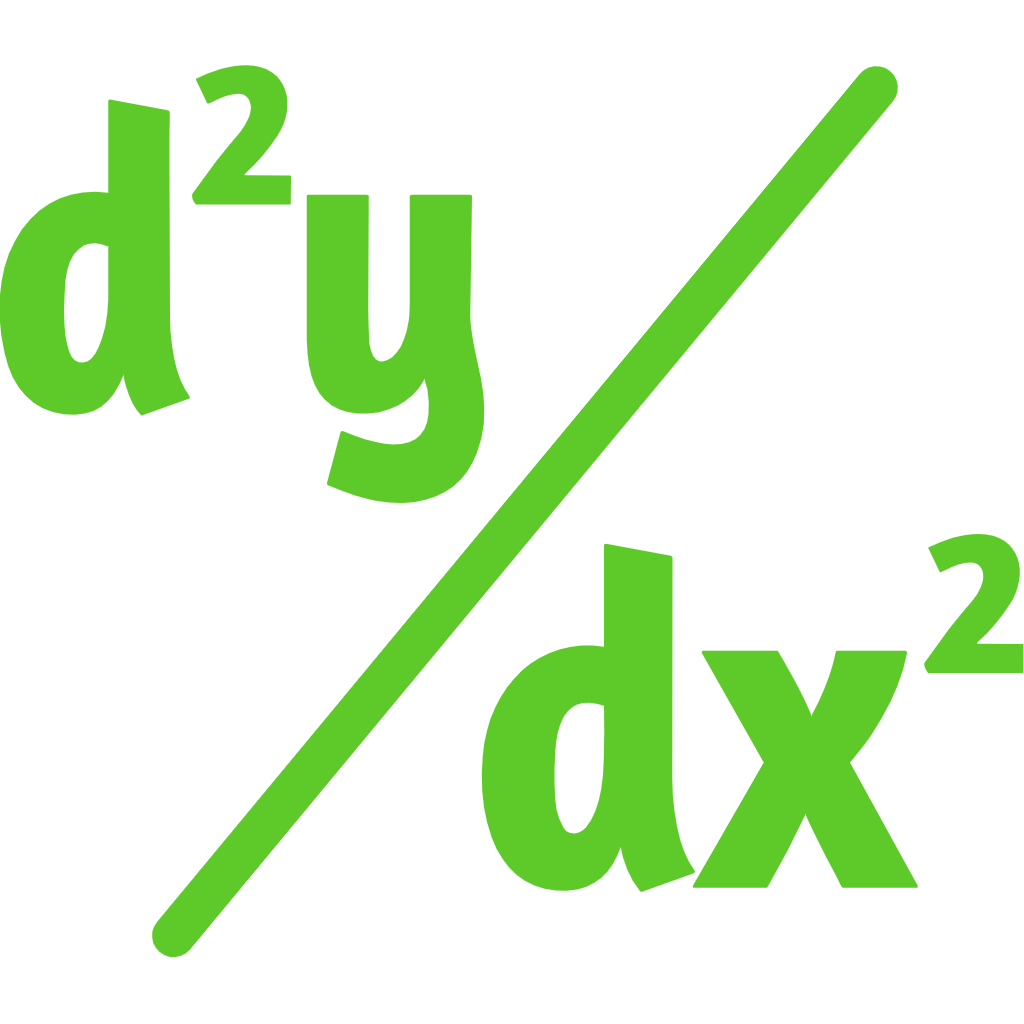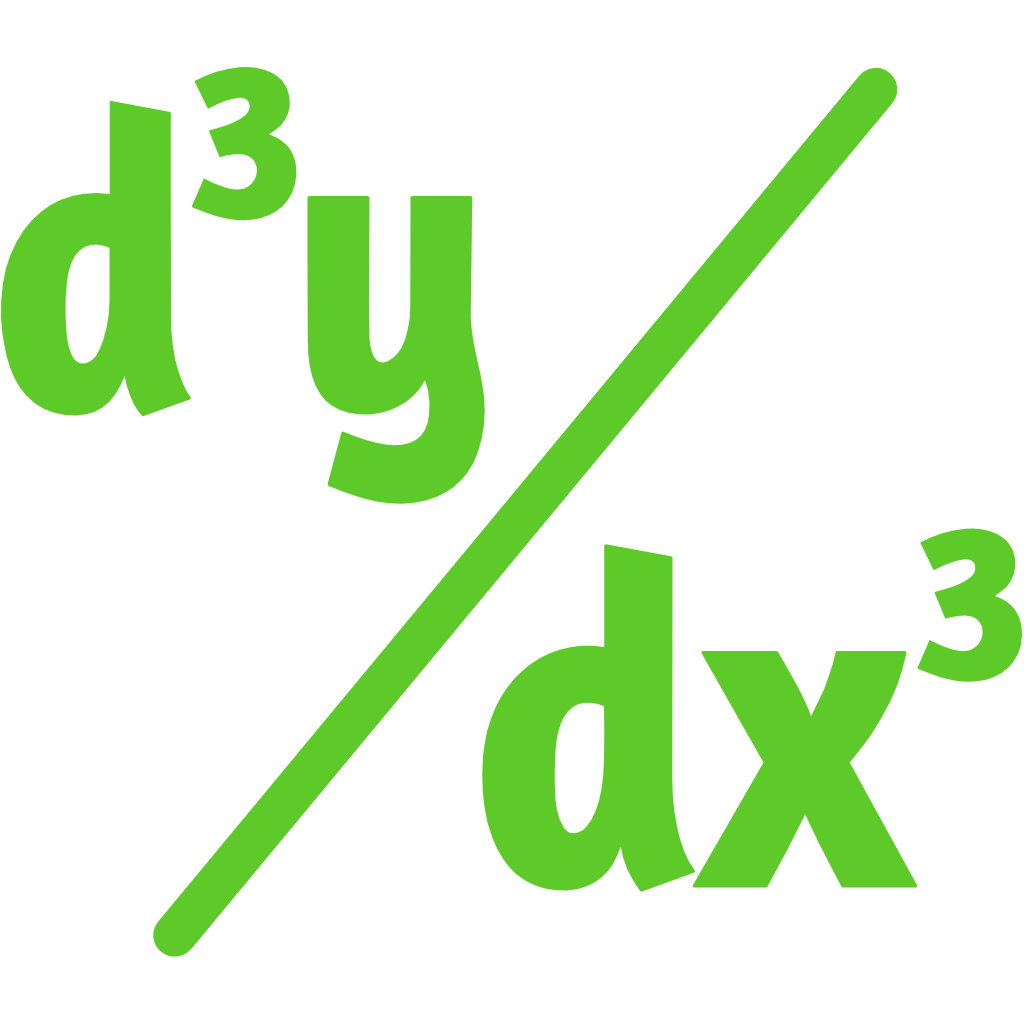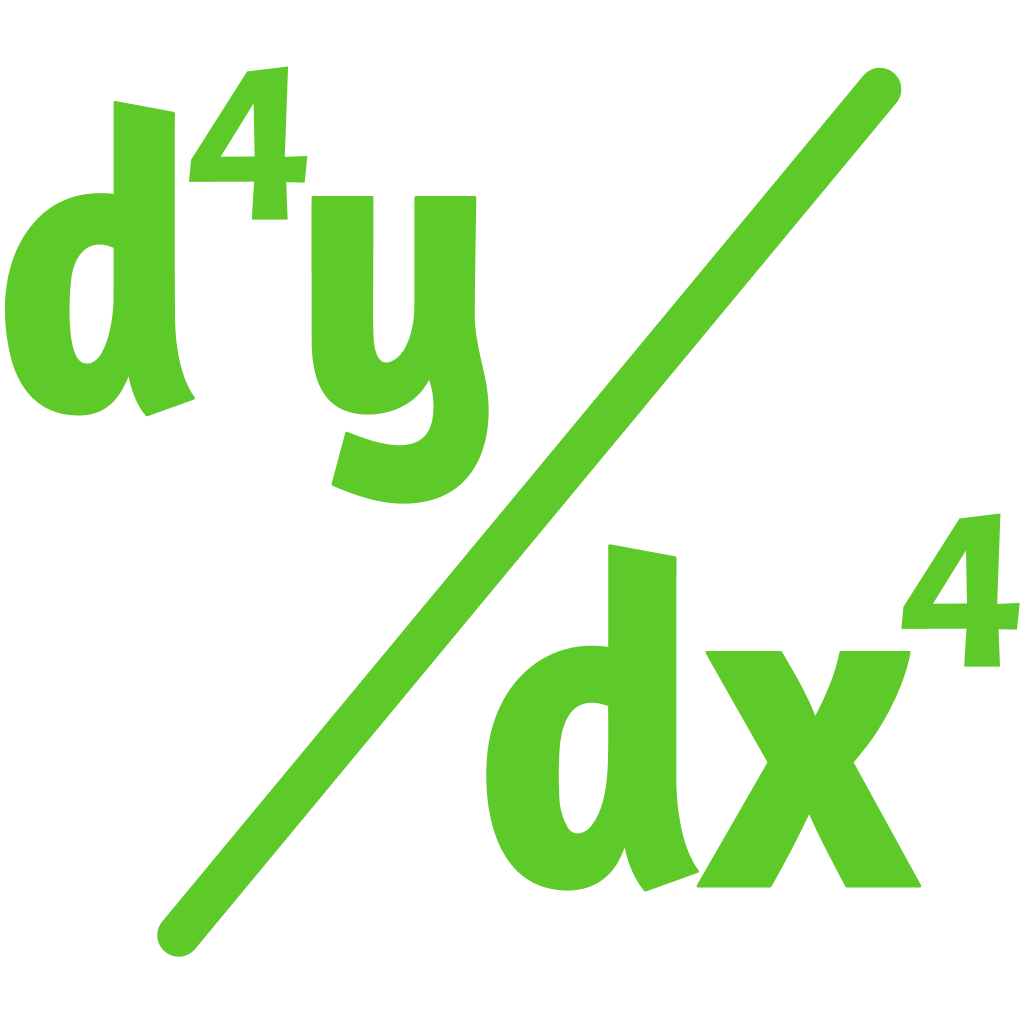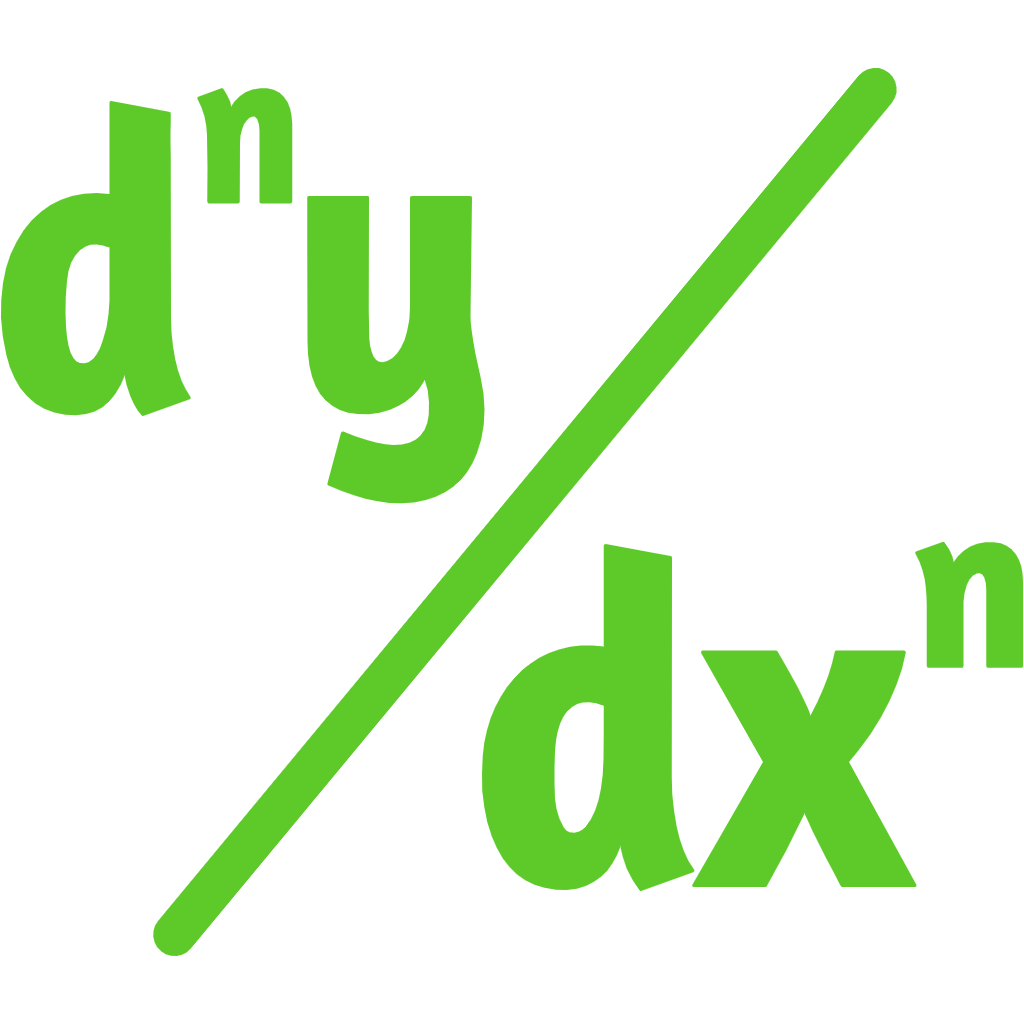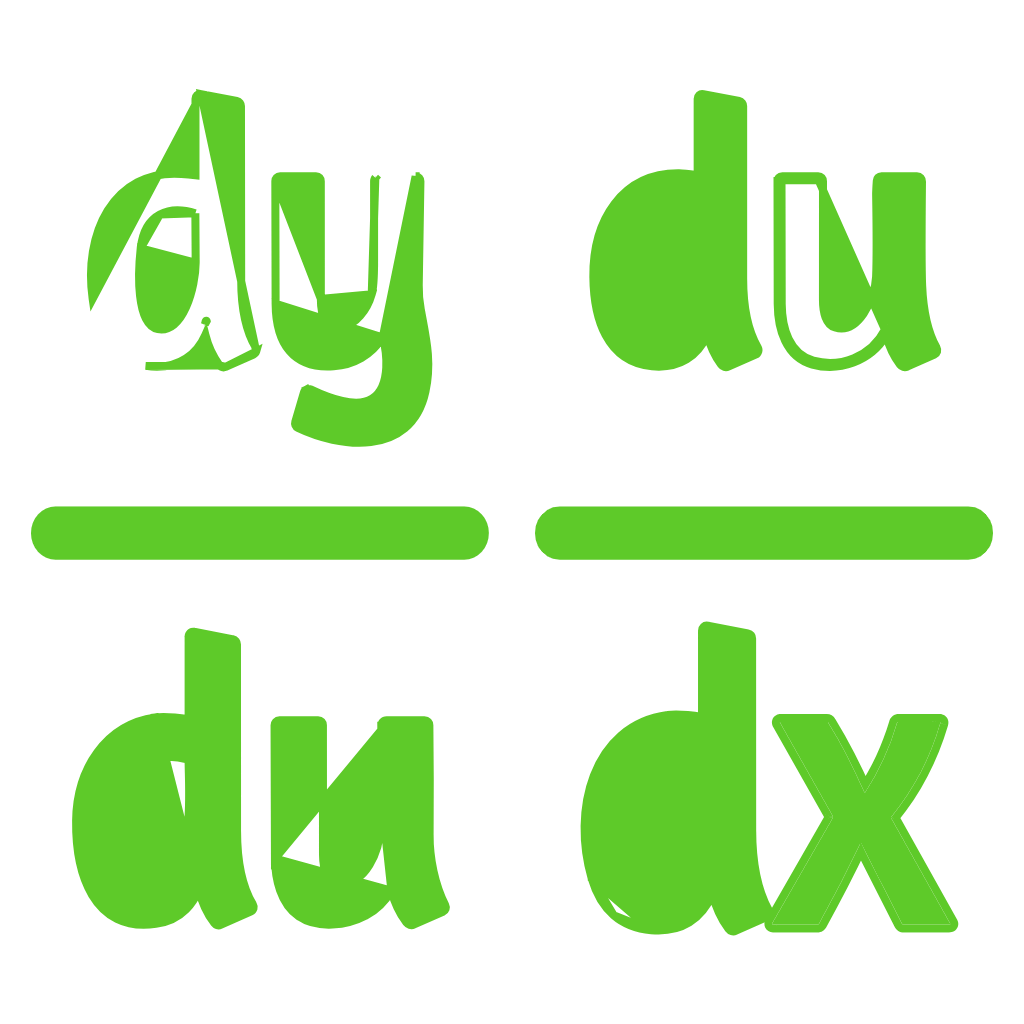Introduction to the Slope of the Curve Calculator
The slope of a curve calculator is an online tool that helps to find the equation of a normal line. Our calculator provides an easy method to calculate the slope of a tangent line of the curve.
For calculus students, it is a beneficial tool for complicated trigonometric problems of the slope of a curve and gives instinctive results.
our Slope of the Curve Calculator offers users a seamless experience in evaluating slopes for various types of functions. Whether dealing with polynomial functions, trigonometric functions, exponential functions, or any other mathematical expression, our calculator can handle them all.
Additionally, if you would like to evaluate a function derivative at a given point, you can use our derivative at a point caluclator. Our calculator also to compute the average rate of change of the differential function at a particular point.
What is the Curve Line Slope?
The Curved line slope is defined as a point where a normal line is formed on a slope of the curve. It is an important concept in mathematics. There is no specific formula to calculate the curve line slope but our calculator can find it by some steps.
The slope of the curve, derived from the concept of derivatives, provides practical insights into the behavior of functions. By using the Definition of Derivative Calculator to understand the theoretical underpinnings of derivatives, users can apply this knowledge to calculate slopes of curves and interpret their significance in real-world scenarios.
Evaluation Process of the Slope of a Curve Calculator
The slope of a curve at a point calculator computes the instantaneous rate of change of the curve at a point of a tangent. To find the curved line slope you just enter your problem with coordinates values (x,y) and a calculator will automatically do the remaining task.
After taking the required values, the curved line slope calculator takes the derivative of function y` and puts the given point value of x,y in this linear equation y=mx+c. At last, add point c in the given function to give the solution.
With that, the slope of the curve calculator provides the solution in the graph that helps you to understand the curved line slope concept easily.
Additionally, for further exploration of function behavior and graphical analysis, you can be go through our Derivative Graph Calculator. This calculator enables users to visualize the derivative graph of a function, providing insights into its rate of change and critical points.
Solved Example of Curved Line Slope
Now let's visualize an example of a curved line slope. Although curved line slope can be calculated using a slope of the curve calculator but it can also be done manually. An example of the manual calculations is given below,
Example:
Find the following:
$$ y \;=\; sin(x) cos(x), (2,4) $$
Solution:
Find the tangent line equation:
$$ y(x) \;=\; sin(x) cos(x) \;at\; x_0 \;=\; 2 $$
Compute the derivative of y(x), which will be used to find the slope of the tangent line:
$$ y’(x) \;=\; cos^2 (x) - sin^2 (x) $$
Substituting x0 into y’(x):
$$ m \;=\; y’(x_0) \;=\; cos^2 (2) - sin^2 (2) $$
Evaluate the equation:
$$ y_0 \;=\; y(x_0) \;=\; cos(2) sin(2) $$
Now write the equation in the form of y = mx + y(0):
$$ y \;=\; cos(4) x - 2 cos(4) + cos(2) sin(2) $$
Thus it is the final solution of our function with specific limits. Additionally, for further analysis and computation of second implicit derivatives, you can utilize our Second Implicit Derivative Calculator. This calculator provides a convenient way to compute the second derivative of implicitly defined functions, offering insights into the curvature and behavior of the curve.
How to Use the Slope of the Curve Calculator?
This slope of a curve calculator is a free tool used to find the slope of the tangent of a curve. So you can get an accurate solution to the curve line slope problems by following these steps:
- Enter the curved line slope function f(x) in its respective field
- Enter the x number value in the x coordinate box.
- Enter the y number value in the y coordinate box.
- Click on the "Calculate" button to get the solution of a curved line.
Additionally, for further exploration of derivative concepts and functions, you sholud be use our Implicit Differentiation Calculator. This calculator facilitates the computation of derivatives for functions defined implicitly, expanding your capabilities in function analysis and problem-solving.
Result Obtained from Slope of Curve Calculator
You will get a result within a minute after entering the input of a curved line problem in the slope of the curve calculator. You will see some options like:
- In the first box, a result of the given problem
- Possible step solution section will give you a detailed explanation
- The plot option will give you a graphical representation of your problem.
- Click on “Recalculate” for new calculation of more examples
Related:For further exploration of derivative concepts and function, you can use our Inverse Derivative Calculator. This calculator facilitates the computation of derivatives for inverse functions, providing valuable insights into function behavior and mathematical analysis.
Why do We Need the Slope of a Curve at a Point Calculator?
The curved line slope calculator will give the solution of a steepness line at a constant term and the direction of the curve on the coordinate plane (x,y) like the velocity of a particle within a fluid.
While calculating the Slope of a curved line manually, you may need help in complex calculations or may have made any mistakes.
To remove errors in calculation we introduce our slope of the curve calculator which is helpful to solve any type of function to avoid any mistakes in the solution. You will easily understand the concept from this slope of a curve calculator, especially for those who want to learn the curved line slope concept without any tutor.
To delve deeper into advanced calculus concepts and techniques, you can utilize the Logarithmic Differentiation Calculator. This calculator employs logarithmic differentiation methods to differentiate complex functions, providing step-by-step solutions for enhanced learning and understanding.
By leveraging the Slope of a Curve at a Point Calculator and other mathematical tools, you can enhance your understanding of curved lines and their slopes, paving the way for more informed analysis and problem-solving.
Benefits of Using Our Curved Line Slope Calculator
The slope of curve calculator will provide tons of benefits for complex functions with graphical representation.
- It has a simple interface which means it provides an easy-to-use procedure for beginners
- This slope of a curve at a point calculator is a free online tool that does not require a subscription fee.
- Our calculator allows you to calculate various types of functions.
- It saves time from doing complicated problem evaluation
- The slope of the curve calculator is a reliable tool that gives accurate results.
- The solution will be detailed with a complete calculation procedure to help a user easily understand its concepts.
For a comprehensive collection of calculators catering to various mathematical needs, including slope determination, consider exploring our All Calculator. This centralized hub provides access to a range of calculators, offering solutions for diverse mathematical tasks and scenarios.