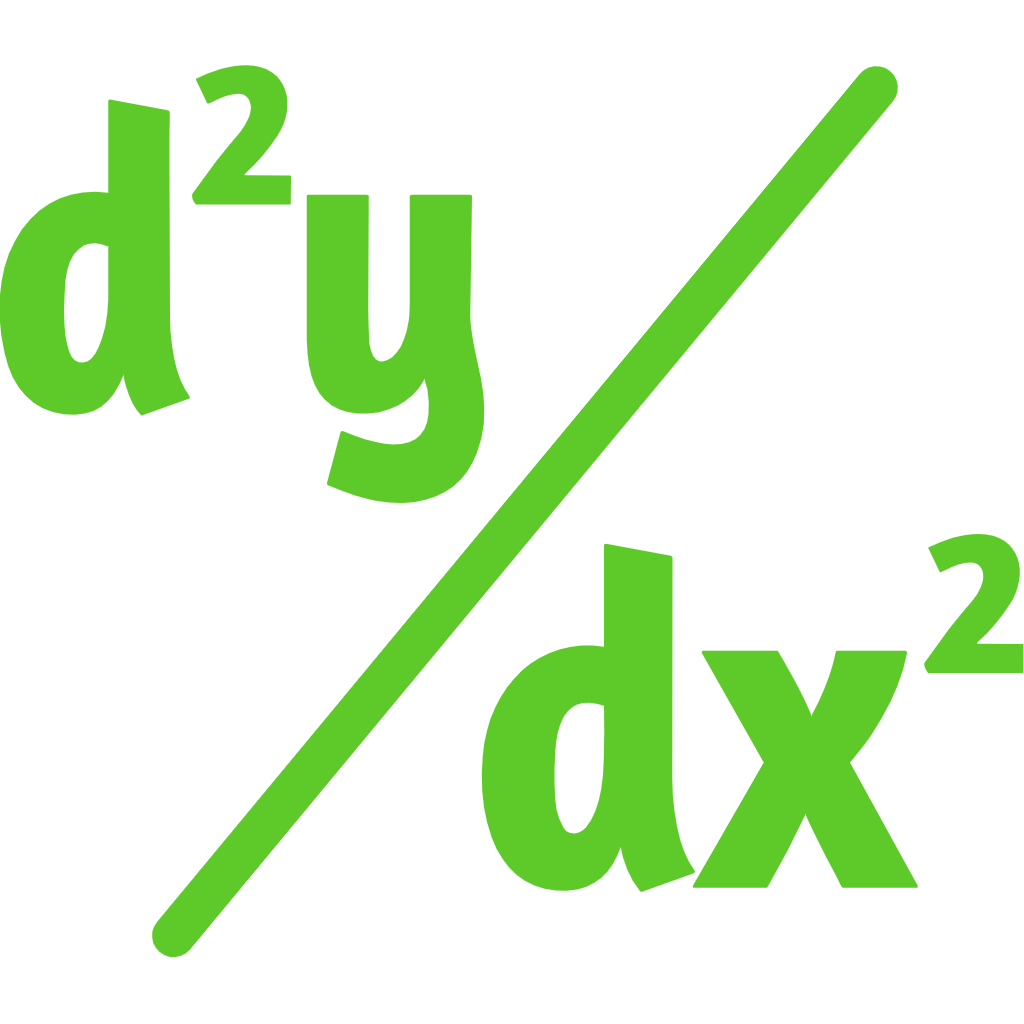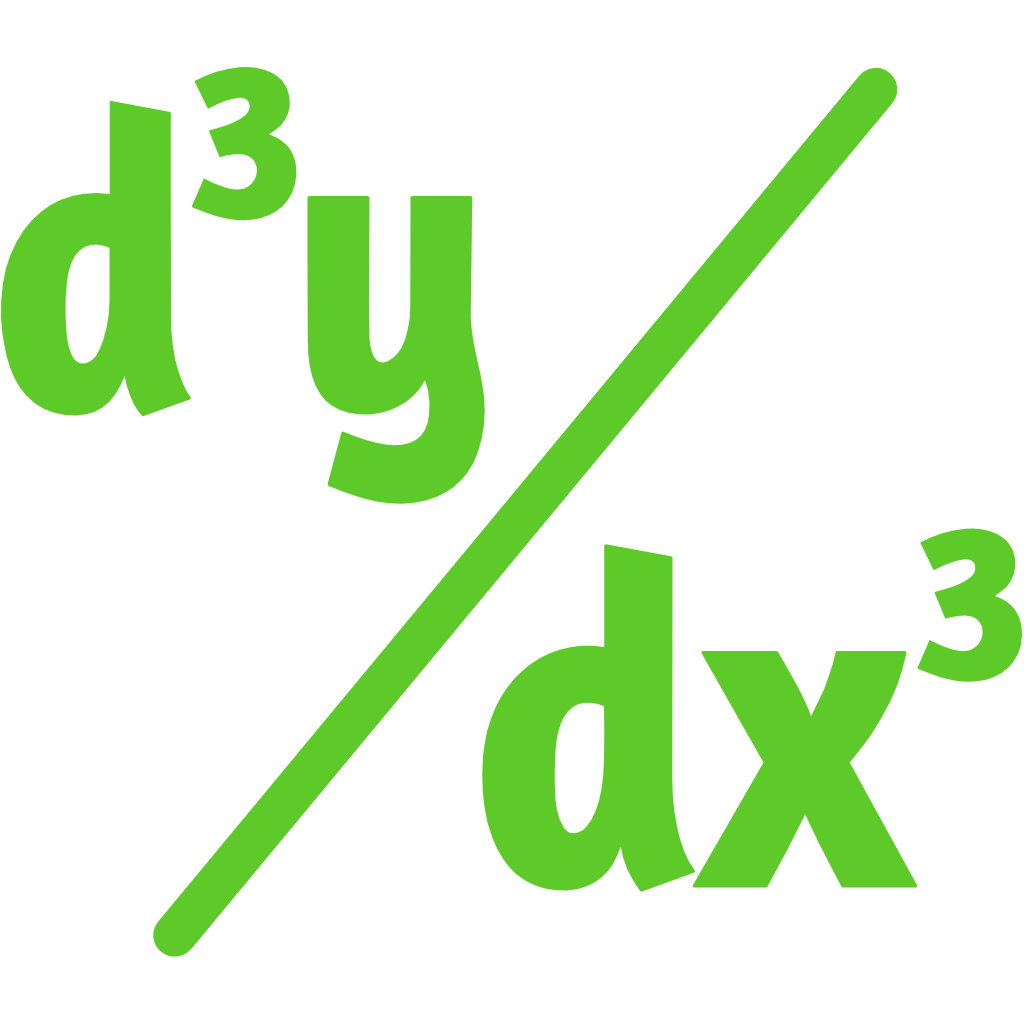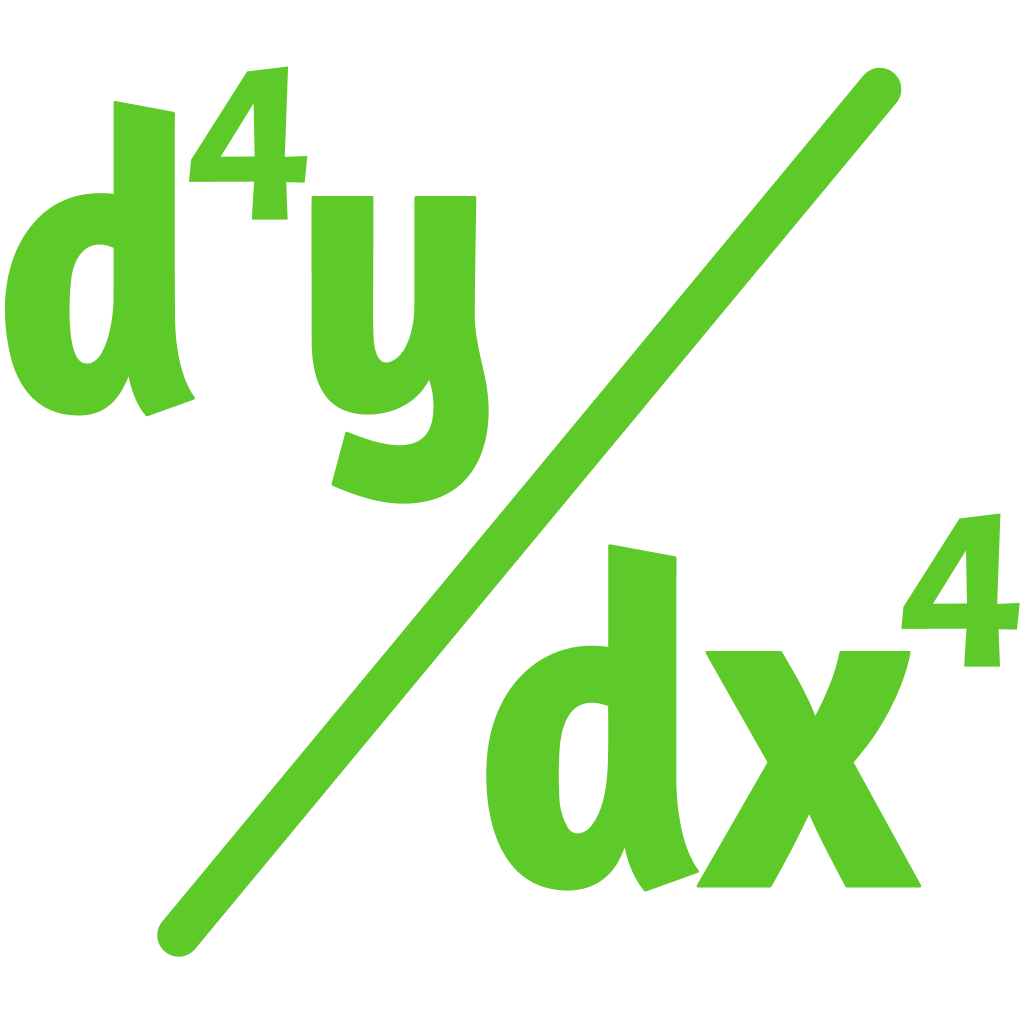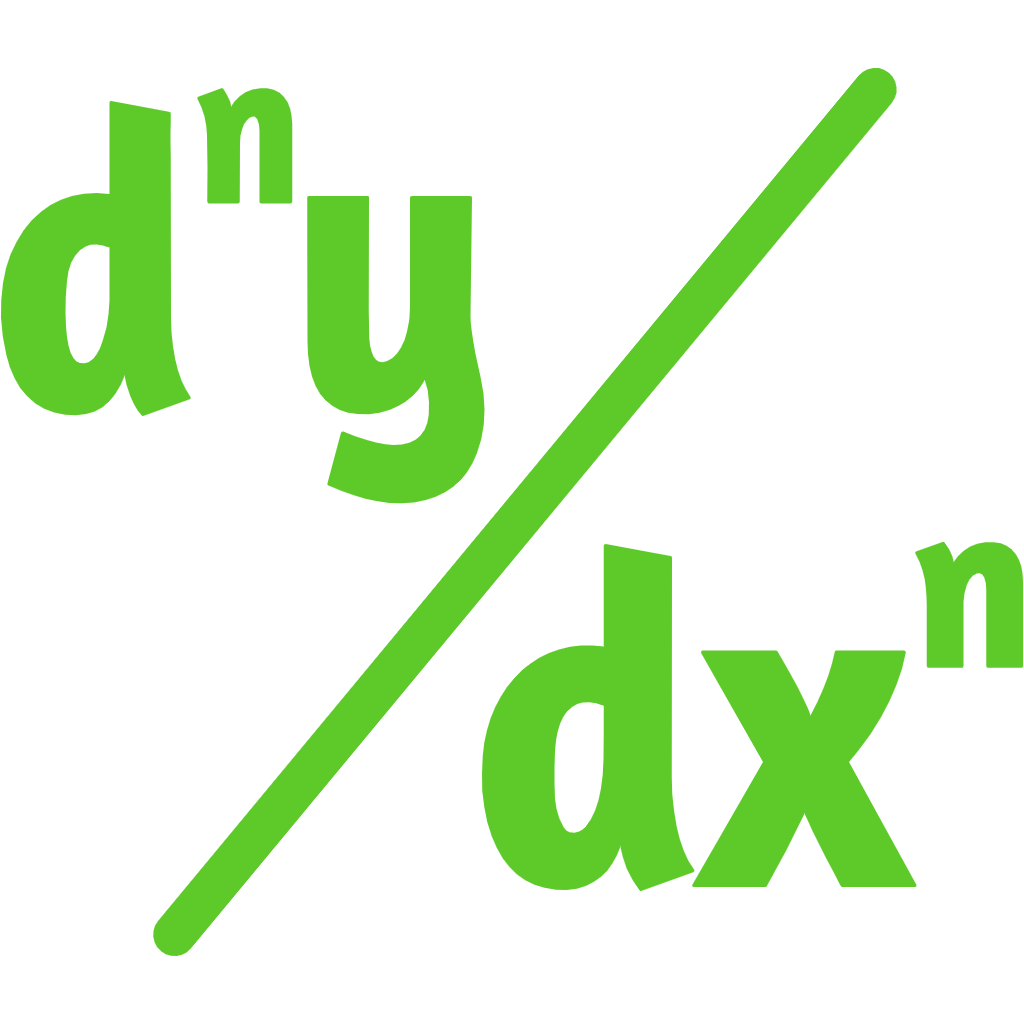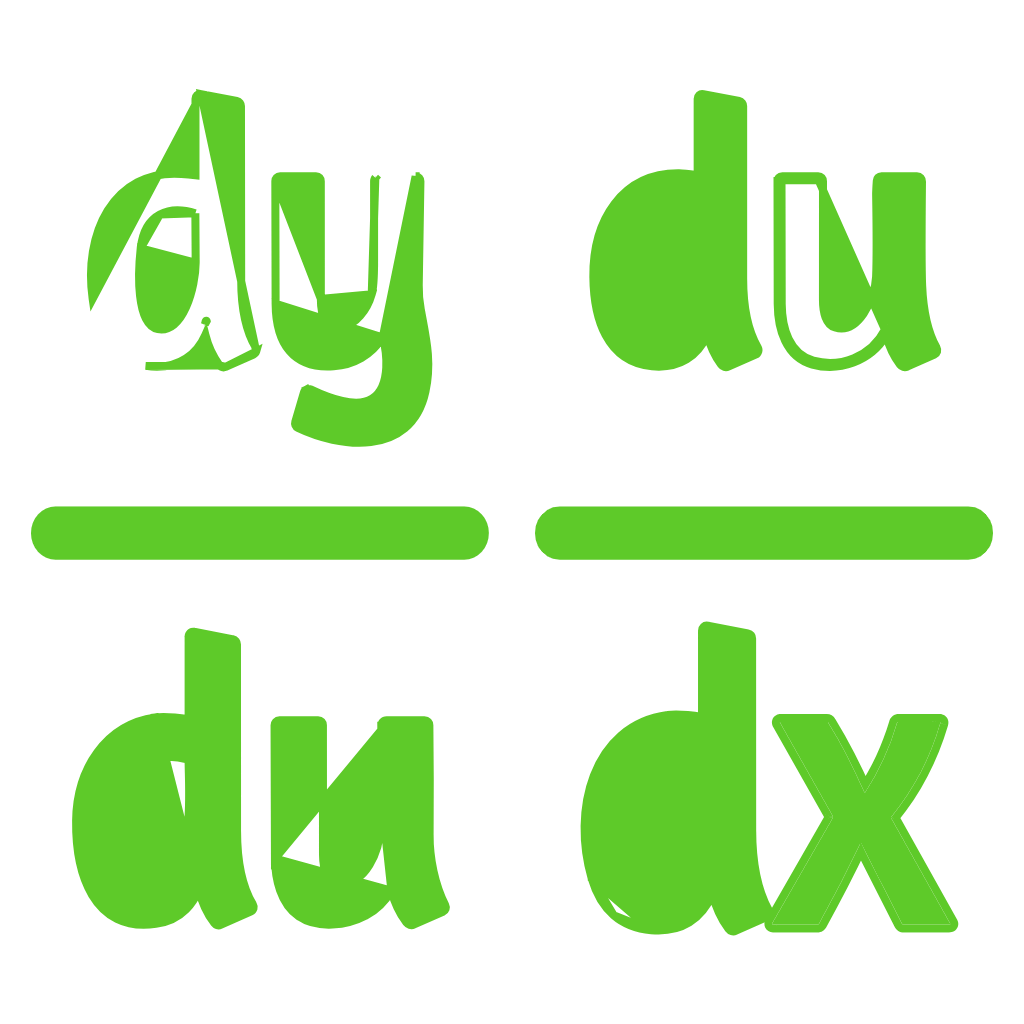Introduction to Maclaurin Series Calculator with Steps
The Maclaurin polynomial calculator is a mathematical tool that shows the expansion of the Maclaurin series for a function at a fixed point. It is used to find a function that has Taylor series expansion of the sum of derivatives of a given function.
Maclaurin calculator is a valuable tool for students, and researchers who want this type of calculator to find complex Maclaurin series functions with a detailed procedure in the steps for a better understanding.
Additionall, for computations involving Taylor series expansions centered at arbitrary points, you can go through our taylor's polynomial calculator. This versatile calculator computes Taylor series expansions for functions around specified points, offering a broader range of applications in mathematical analysis.
What is a Maclaurin Series?
Maclaurin series is the expanded form of the Taylor series of the function at fixed points It is called the Maclaurin series. This series is derived from the Taylor series.
The Maclaurin series provides a polynomial approximation of a function around the point x=0. By including higher-order terms in the series expansion, the approximation becomes increasingly accurate within a certain interval around the origin.
while the Maclaurin Series Calculator focuses on expansions around x=0 in real analysis, whereas the laurent series calculator handles expansions around complex points in complex analysis.
Equation used by Maclaurin Polynomial Calculator
The equation of the Maclaurin Series behind the Maclaurin series calculator is given,
$$ f(x) \;=\; \sum_{n=0}^{\infty} \frac{f^{(n)} (0)}{n!} x^n \;=\; f(0) + f’(0)x + \frac{f’’(0)}{2!} x^2 + \frac{f’’’(0)}{3!} x^3 + … + \frac{f^(n) (0)}{n!} x^n + … $$
Maclaurin Series has a fixed point in the Taylor Series f(x) which is a=0 then f(a).
This equation provides a polynomial approximation of the function f(x) around the point x=0, known as the Maclaurin series expansion.
Related:For further exploration into mathematical series, consider using our Puiseux Series Calculator, providing tools for analyzing Puiseux series expansions and related concepts.
Working Method of Maclaurin Calculator
Maclaurin expansion calculator has a series expansion formula and the basic rules of derivatives, factorials as well as arithmetic and geometric functions in its server for Maclaurin series solution.
That means you do not need to put a lot of effort into getting accurate solutions.
When you enter your function in the maclaurin series expansion calculator it applies the derivation rule to the given function after identifying the nature of the given function.
McLaurin series calculator differentiates the function in n several times and then puts a=0 a fixed point in derivation(first, second, third, or even number of derivatives) as f(a). Then add these numbers of derivatives in the form of a series. With that, you will get solutions with steps that further provide you with simplification and clarity about the maclurin series concept.
Further, for computations involving gradients and directional derivatives of multivariable functions, you would like to use our gradient calculator. Our calculator computes gradients and provides directional derivatives, facilitating the analysis of vector fields and scalar fields in multivariable calculus.
An example of the Maclaurin series is given to help you understand the workings of the calculator.
Example of Maclaurin Series
Maclaurin series can be determined by using the Maclaurin series calculator with steps but it is also essential to solve it manually. So here is a manual example of the Maclaurin series with steps, where each step is explained in detail.
Example:
Find the Maclaurin series for the function f(x) = sin(x):
Solution:
$$ f(0) \;=\; sin(0) \;=\; 0 $$
$$ f’(x) \;=\; cos(x) . f’(0) \;=\; cos0 \;=\; 1 $$
$$ f’’(x) \;=\; sin(x) . f’’(0) \;=\; -sin(0) \;=\; 0 $$
$$ f’’’(x) \;=\; -cos(0) . f’’’(0) \;=\; -cos(0) \;=\; -1 $$
Now write the Maclaurin series :
$$ sin(x) \;=\; 0 + (1) x + \frac{0}{2!} x^2 + \frac{-1}{3!} x^3 + … $$
Simplify:
$$ sin(x) \;=\; x - \frac{x^3}{6} $$
$$ sin(x) \;=\; \sum_{n = 0}^{\infty} \frac{(-1)^k}{(2k + 1)!} x^{2k + 1} $$
This represents the sine function as an infinite alternating sum of terms which involves the odd powers of x.
Related:For further exploration of calculus concepts, including advanced topics like vector calculus, you can use our curl calculator.
How to Use the Maclaurin Series Calculator
Maclaurin polynomial calculator has a user-friendly interface that provides you with given Maclaurin series results in no time. You need to follow some instructions that are given below while using this maclaurin calculator.
- Enter your given Maclaurin function in the respective field.
- Enter the number of orders in the relevant box.
- Choose the variable which you want to differentiate the function from the list.
- Click on the calculate button to get the expansion of the series
Additionally, for computations involving divergence and vector fields in vector calculus, you can use our divergence calculator. This calculator calculates the divergence of vector fields, aiding in the analysis of fluid flow, electromagnetism, and more.
Result Obtained from Maclaurin Expansion Calculator
You will get the result of the given function instantly after adding the input value to the McLaurin series calculator. It may include as:
- Solution section will show the answer to the given problem
- Possible steps section give you the solution of the Maclaurin series in detail
- Recalculate button provides a new page for further evaluation of the Maclaurin series.
Why Use our Maclaurin Series Expansion Calculator?
Maclaurin series calculator with steps is a versatile tool as it can solve various types of questions like exponential, derivative, infinite series, etc in the run of time with minimal error.
This Maclaurin polynomial calculator will allow you to change your function values according to the expression of a question which keeps you away from the trouble of doing lengthy calculations of different functions.
If a person is doing calculations by hand it takes a lot of time because the Maclaurin series has complicated procedures. Our Maclaurin calculator provides a speedy calculation of the Maclaurin function in no second. It is a certificate tool by renowned university professors and mathematicians.
For further analysis of extrema in functions, you can use our extrema calculator. This tool computes critical points, local and global extrema, aiding in the optimization and analysis of functions.
Benefits of the Mclaurin Series Calculator
This Maclaurin expansion calculator will provide you with multiple benefits as it will give solutions to all types of the Maclaurin series. These benefits are:
- It saves time and human effort that you put into the calculation of the Maclaurin series.
- It has a simple design so that anyone can use it easily
- The Maclaurin series expansion calculator gives you an accurate solution with steps of the Maclaurin problem.
- You can use it for practice questions to get command of this concept
- Our Maclaurin series calculator is so a handy tool as it used from any electronic device through the internet
Further, explore the Maclaurin Series Calculator and other mathematical tools available in our collection by visiting the All Calculators section. Whether you need to compute series expansions, solve equations, or analyze functions, our comprehensive range of calculators has you covered for all your mathematical needs.