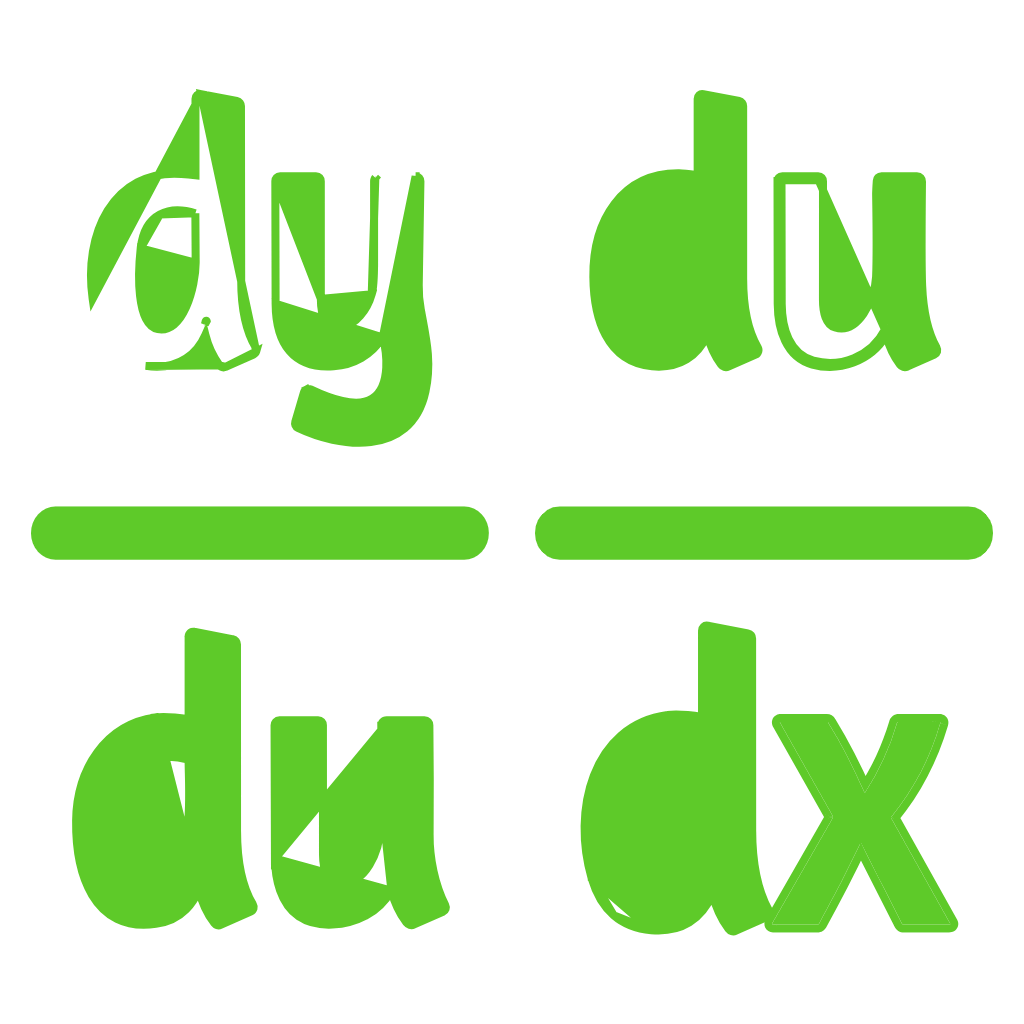Introduction to Puiseux Series Calculator
The Puiseux series calculator is an online tool that is used to find the Power series of complex analysis. It evaluates the fractional exponential of a polynomial in a bounded field of nth root in a run of time.
Our Puiseux Series Calculator offers you a convenient and efficient way to compute Puiseux series expansions, providing valuable insights into the behavior of functions near singular points. Whether you're a student, researcher, or professional, our calculator is a valuable tool for exploring the intricacies of algebraic singularities and advancing your understanding of mathematical concepts.
Additionally, for computations involving smooth functions and local approximations, consider using our taylor series expansion calculator. This calculator is designed to compute Taylor series expansions, providing insights into function behavior around specific points and facilitating local approximations for a wide range of functions.
Definition of Puiseux Series
A Puiseux series is a power series that contains non-negative fractional exponents and logarithms, where the logarithms multiply with a polynomial fixed. It includes the denominators of the exponents that are bounded.
When you reduce the exponents to a common denominator n root, the series becomes a Laurent series in an nth root of the indeterminate. In the Puiseux series, a complex number has n nth roots, and it converges the Puiseux series into n functions in a neighborhood of 0.
Additionally, for practical applications and to understand the relationship between Puiseux series and Laurent series, you can use our laurent series calculator. Our calculator also evaluates the analytic functions whose initial point is given and also provides the series of convergence in solution in a few seconds.
Formula of Puiseux Series
Puiseux series expansion depends on power series in a bounded region of the field. The notation used by Puiseux series calculator is,
$$ x(t) \;=\; \sum_{i=i_0}^{\infty } c_i t^{\frac{1}{m}, i_0, m \in \mathbb{Z}, m \gt 0, c_i \in \mathbb{C} $$
Whereas,
- x(t) is the Puiseux series in t
- t 1/m = is the Laurent series
- Puiseux series is bounded field k,k>0
- K{t} = denote Puiseux series field
Additionally, for computations involving power series expansions centered at zero, you can use our maclaurin calculator. This calculator specializes in computing Maclaurin series expansions, providing insights into the behavior of functions around the origin and facilitating local approximations for a wide range of functions.
Working Procedure of Our Calculator
Our calculator uses the power series expansion method to solve various types of complex series functions. Its main reason is that our calculator is equipped with advanced mathematics features that give solutions in a couple of times.
When you add the input value function in the puiseux series calculator, it will analyze the series then as per the nature of the function. Then the given function is expanded according to the Puiseux series expansion in a bounded field.
Puiseux series converges when k is greater than zero as k>1. Sometimes you do not need to apply Puiseux power series expansion because the exponential fraction can be solved with the Laurent series or with Taylor series expansion easily.
Let's observe an example of the puiseux series for better clarity about this concept. To explore further mathematical tools, consider using our gradient function calculator, which provides insights into gradient computations and related concepts.
Example of Puiseux Series:
An example of puiseux series expansion is given below:
Example:
Use puiseries series of the following:
$$ \sqrt{2} \sqrt{x-1} $$
Solution:
Puiseux series near 1 is
$$ \sqrt{2} \sqrt{x-1} $$
More terms are:
$$ \sqrt{x^2 - 1} \;=\; \sqrt{2}(x-1)^{\frac{1}{2}} + \frac{1}{4} \sqrt{2}(x-1)^{\frac{3}{2}} - \frac{1}{32} \sqrt{2}(x-1)^{\frac{5}{2}} + \frac{\sqrt{2}(x-1)^{\frac{7}{2}}}{128} - \frac{\sqrt{2}[5](x-1)^{\frac{9}{2}}}{2048} + \frac{\sqrt{2}[7](x-1)^{\frac{11}{2}}}{8192} + …. $$
Additionally, for more advanced mathematical computations and insights, you can go through our vector curl calculator, which provides tools for calculating curl operations and related concepts.
Using the Puiseux Series Calculator
The Puiseux series tool is an easy-to-use tool as it has a user-friendly interface that provides its users with a comfortable experience that enables them to use it for the evaluation of exponential power series problems. You need to follow some steps before calculation. These steps are:
- Enter the power series function in its respective field
- Add the point if it is not zero in the input field
- Click on the calculate button to get the solution of the Puiseux series expansion
- Review your power series function before pressing the calculate button.
- Press the recalculate button that brings you back to the home page where you can do more evaluation of puiseux series problems.
- You must try our calculator to observe its accuracy in solutions using our load examples.
Related:For computations involving the analysis of divergence of sequences or series, consider using our divergence of vector field calculator. This calculator assists in determining the convergence or divergence of sequences and series, providing insights into their behavior and properties.
Final Result from Our Calculator
You get the final result from the Puiseux series calculator immediately after you enter the input of the power series of functions in it. It may include as
- Result option
Result option provides you solution of the power series of puiseux series expansion
- Possible steps
Steps option gives the solution of puiseux series in detail
- Plot option
This option draws a graph after taking the solution values from the puiseux series solution.
Moreover, for computations involving the analysis of extrema (maximum and minimum points) of functions, you can utilize our extreme value calculator. This calculator assists in identifying critical points, determining their nature, and analyzing the behavior of functions near extrema.
Advantages of Using the Calculator
The calculator gives you multiple benefits whenever you give the input value in it and get the result. You just need to put your power series function and the rest of the calculation will be done in the calculator in a few seconds. These benefits are:
- Our tool keeps you away from doing lengthy calculations of power series questions
- The calculator has a simple design so everyone can manage it easily for series expansion.
- You do not need to spend a single money for the calculation of complex functions in it.
- It is a trustworthy tool that always gives you accurate solutions for your given function without any errors.
- It is a speedy calculator that provides a solution for a power series of puiseux expansion in a few seconds
- You can use it to solve different types of complex series functions using our tool.
- The Puiseux series calculator provides solutions in the form of a graph for better understanding visually.
Experience the benefits of our Laurent Series Expansion Calculator and explore a wealth of mathematical tools on our All Calculators page. Simplify your computations and elevate your mathematical prowess with ease.