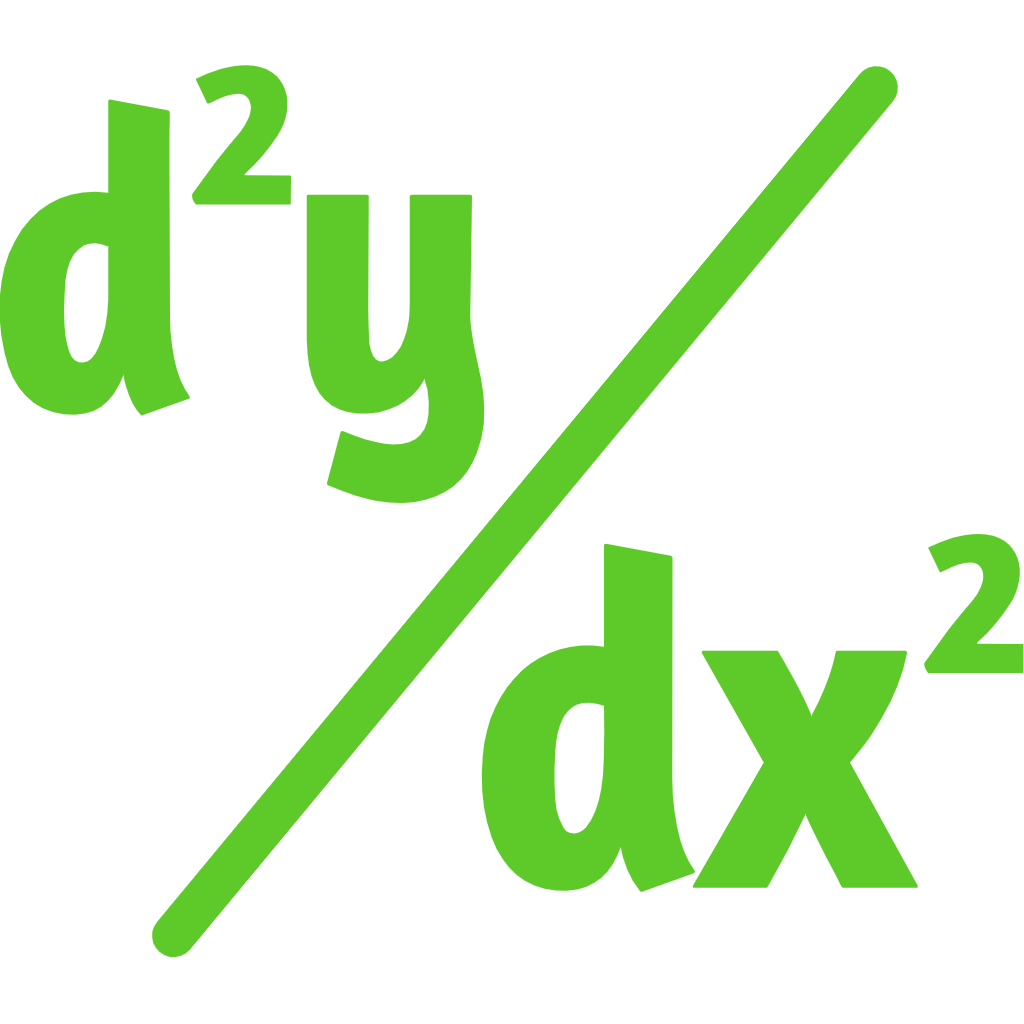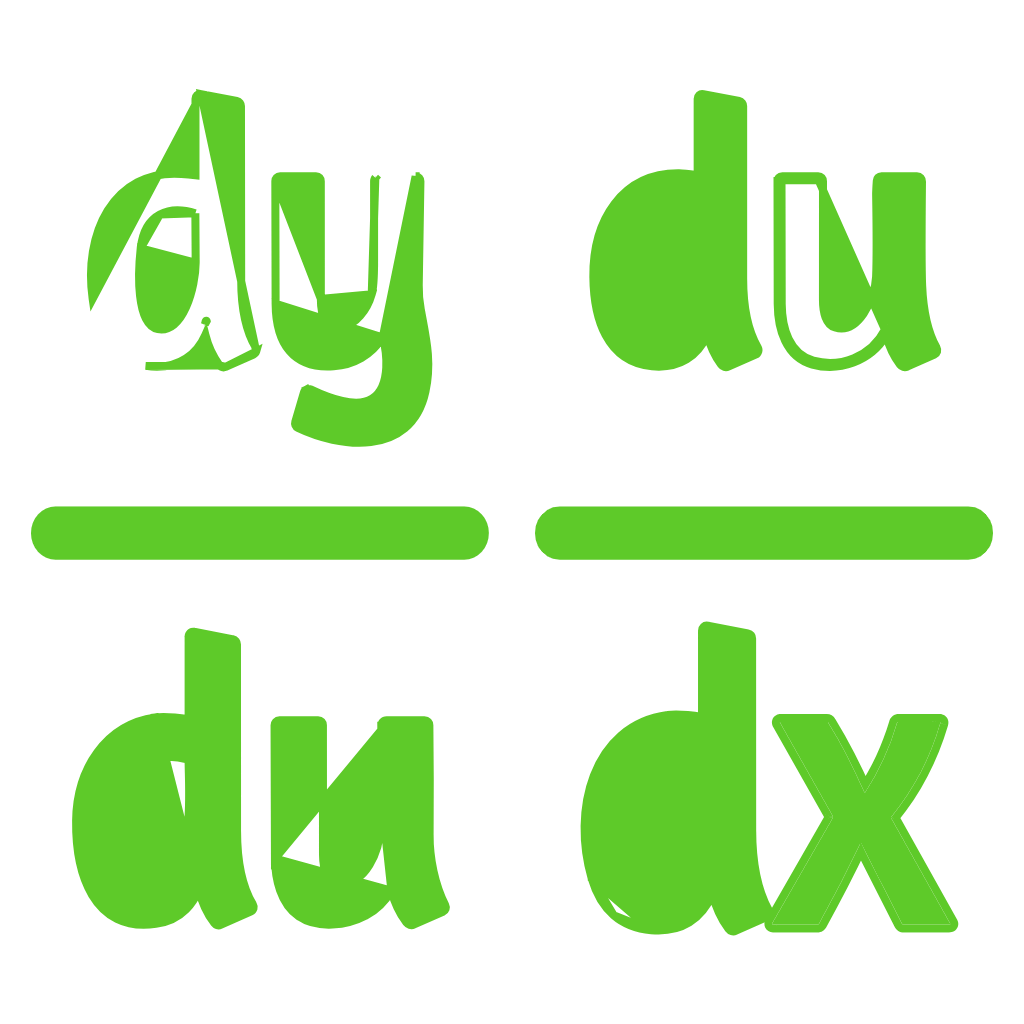Introduction to the Implicit Differentiation Calculator with Steps
The dy/dx calculator is an online mathematical tool that evaluates derivative function in terms of x and y both variables. It provides solutions to differential problems with respect to dependent variables in detail in a run of time.
An implicit differentiation is a tool that helps you compute the derivative of an implicitly defined function while showing the step-by-step process. This can be extremely helpful for students and professionals alike who are learning or applying implicit differentiation in calculus.
implicit differentiation calculator with steps is a valuable tool for learning and practicing implicit differentiation, as it provides immediate feedback and guidance on the differentiation process.
Additionally, if you would like to find the derivative of a second-order implicit function, you can utilize our d2y/dx2 calculator. This calculator also useful for analyzing the concavity, inflection points, and other curvature properties of curves defined implicitly.
What is an Implicit Derivative?
Implicit differentiation is a process of finding the derivative of both dependent and independent variables because it does not have one specific independent variable for differentiation as shown below,
$$ x^2 + y^2 \;=\; 1 $$
$$ \frac{d}{dx} \biggr( x^2 + y^2 \biggr) \;=\; \frac{d}{dx} (1) $$
In calculus, when you have an equation where one variable is implicitly defined in terms of another variable, you can use implicit differentiation to find the derivative of the function with respect to the independent variable.
Our calculator evaluates derivative function in terms of x and y both variables. Additionally, if you would like to evaluate a function derivative at a given point, you can use our derivative calculator at a point, This calculator also allows you to compute the average rate of change of the differential function at a particular point.
Working Procedure dy/dx Calculator with Steps
Our implicit differentiation calculator is used to compute the function when a given problem does not specify the independent or dependent variable. These types of derivative equations are difficult to solve from normal differentiation, so this tool applies the chain rule for the implicit differential function.
You need to enter your implicit function in this implicit derivative calculator with steps and it will get a solution. When you give the input of an implicit function in the calculator, it analyzes to determine the given function behavior.
After recognizing the given function types, it chooses an independent or dependent variable. Then differentiate the given function with respect to an independent variable by keeping the other variable constant and the solution appears on the screen in a fraction of a second.
Additionally, for visualizing derivative functions and their behavior, you can go through our derivative graph calculator. This calculator plots the graph of a given function and its derivative, providing a graphical representation of their relationship and aiding in the visualization of mathematical concepts.
Now we will discuss an example of a differentiation equation to show how an implicit derivative calculator really works.
Solved Example of the Implicit Derivative Problem
Implicit function can be calculated with the help of our implicit differentiation calculator with steps but its also important to do it manually. Here is an example given so that you may know how to do it.
Example:
Find y’ for xy = 1.
Solution:
Since y is a function of x so,
$$ xy(x) \;=\; 1 $$
$$ y(x) \;=\; \frac{1}{x} $$
Applying derivative,
$$ \frac{d}{dx} \biggr( xy(x) \biggr) \;=\; \frac{d}{dx} 1 $$
$$ 1y(x) + xy(x) \;=\; 0 $$
$$ xy(x) \;=\; -y(x) $$
$$ xy(x) \;=\; -\frac{1}{x} $$
$$ y(x) \;=\; -\frac{1}{x^2} $$
Thus it is the final solution of our function with specific limits. If you would like to quickly find the slope of a curve at a given point, you can use ou our slope of the curve calculator. This tool calculates the slope of a curve at any specified point, helping you analyze the behavior of functions graphically.
How to Evaluate in the Implicit Differentiation Calculator
Our implicit derivative at a point calculator is used to evaluate an implicit differentiation function for a dependent variable only. You just need to follow these simple steps to get the precise solution.
- Enter the implicit function as an input in the respective field.
- Choose the variable from the given list
- Click on the "Calculate" button to get the solution of an implicit derivative problem
Additionally, if you're looking to find the derivative of an inverse function, you can use our inverse derivative calculator. It helps in determining the derivative of the inverse function at a given point, providing valuable insights
Result from the Implicit Derivative Calculator with Steps
When you enter the input of implicit differentiation in this implicit differentiation derivative calculator, it will give you a solution along with some extra options for better clarity.
- Result option will provide you with a solution to your implicit derivative problem
- Possible step section gives you a solution in detail
- Plot section gives you a graphical representation of an implicit function
- Recalculate button gives a clear interface for further solutions.
Why Should you Choose Our Implicit Derivative at a Point Calculator?
Our implicit differentiation calculator with steps is designed in such a way that you can get solutions to complicated implicit functions instantly. Even a new person who does not know derivation rules can understand the solution with steps after just entering the required problem.
It can handle any type of implicit function whether problems related to basic or complex functions. Whether you're dealing with basic functions or complex equations, our calculator can handle them all. You can input any implicit function, and our calculator will compute the derivative accurately.
Get instant results without the need for manual calculations or complex software. Our calculator delivers solutions quickly, saving you time and effort.
Further, If you encounter functions involving logarithms and need to find their derivatives, you can go through our logarithmic differentiation calculator. This Calculator simplifies the process of finding derivatives of complex logarithmic functions, providing step-by-step solutions for better understanding.
Benefits of Implicit Differentiation Derivative Calculator:
The derivative calculator implicit is a very beneficial tool to use for implicit function derivation. It provides multiple benefits during the calculation of implicit functions. These steps are:
- The dy/dx calculator with steps saves time while manually calculating the implicit function
- It has a simple interface anyone can use it easily.
- It provides an accurate solution for complex implicit functions.
- The Implicit derivative calculator with steps helps a user practice more examples of implicit questions online to get a strong grip on these concepts.
- It provides results in detail with an explanation of given implicit problems.
- You don't need any fee to use it as it is a free online implicit derivative at a point calculator.
Related: for further exploration of derivative concepts, you can use our definition of derivative calculator. This calculator assists in understanding the fundamental definition of derivatives and their applications, complementing the functionality of the Implicit Differentiation Derivative Calculator.
Take Care While Giving the Input in the Derivative Calculator Implicit
You should check these mistakes while giving input values in this implicit differentiation calculator, which are given as:
- Enter the correct mathematical structure of the implicit differential function so that no error shows during the calculation process.
- Select the independent variable concerning whom an implicit function for differentiation.
- Review your values before clicking on the calculate button.
Additionally, for a comprehensive collection of mathematical tools and calculators, including the Implicit Differentiation Calculator, explore our All Calculator section. This repository offers a wide array of calculators to cater to diverse mathematical needs and assist you in various computational tasks.