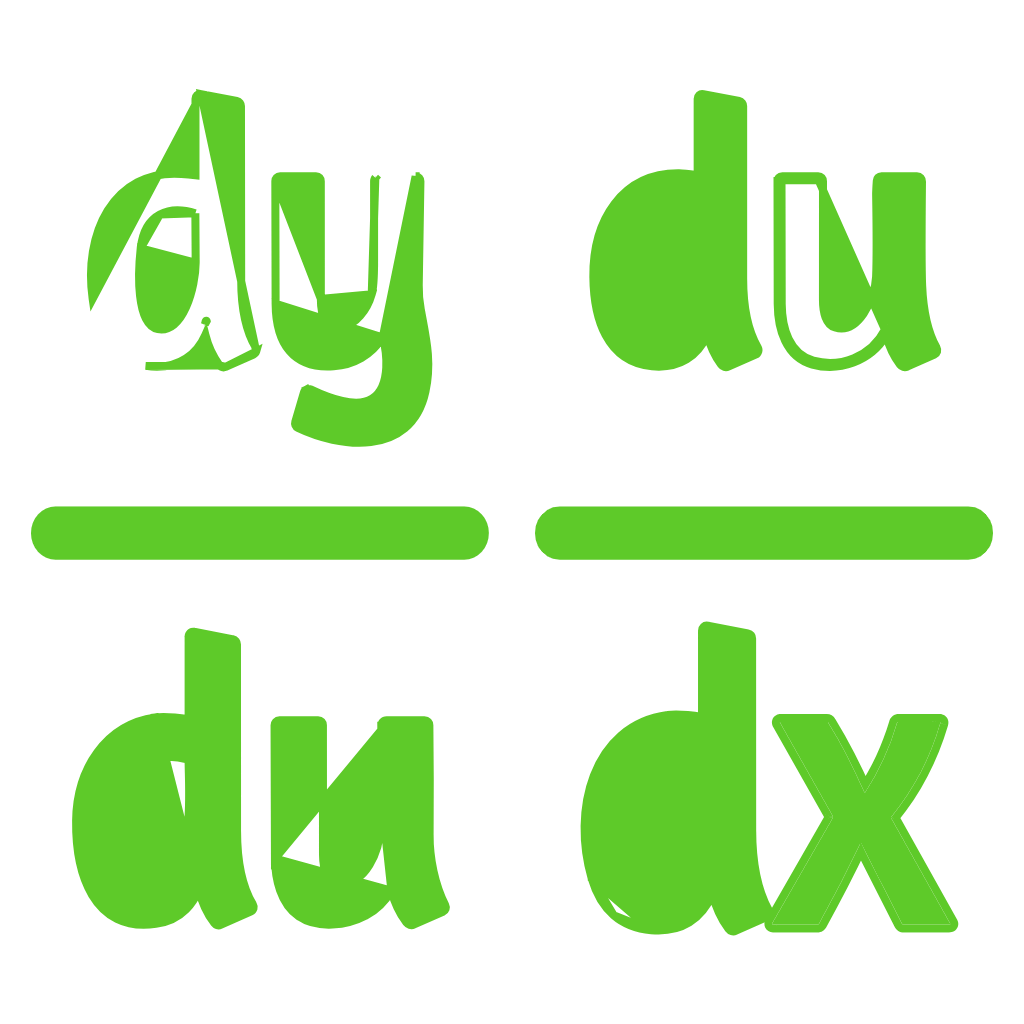Introduction to Local Extrema Calculator
Saddle Point Calculator is an online tool designed to evaluate the critical and extreme points of functions. It provides maxima and minima points of a given function while performing each step of calculations.
To get the solution to mathematical problems related to extreme points we introduce a multivariable tool that helps you to find extreme points of a function. Whether you're a student studying calculus or a professional tackling complex mathematical problems, our Calculator provides a convenient way to identify critical points and understand the behavior of functions within a given interval.
So, our calculator help you find the local extrema of a function quickly and accurately. Further, if you would like to find the curl of vector field functions in two or maybe three dimensions, you can use our curl vector calculator. Our calculator also help to computes the vector function that is rotating about a specific point in one direction in a few seconds.
What is the Extrema Points Value?
An extrema point is a function that gives the largest or smallest values with a particular interval to draw a curve of the function.
In mathematics, the extrema point function uses a derivative process to get information about the rate of change of a curve.
The extrema points, also known as extreme points or critical points, are the values of a function where it reaches its maximum (maximum extrema) or minimum (minimum extrema). These points are crucial in mathematical analysis and optimization problems.
Additionally, if you would like to find the divergence of function in a vector field and provides a solution in the scalar function, you can go through our divergence theorem calculator. Our calculator also help to evaluates the vector field whose flux magnitude is in a directionless system in a fraction of a second.
Evaluation Method of Extreme Points Calculator :
Our calculator works on the basics of the extrema point function. The saddle point calculator is a versatile tool as it can handle all types of complex algebraic functions to find critical points, extrema, absolute minima, and maxima.
When You enter your function in the relative extrema calculator, it checks the nature of the function for derivation. After recognizing the function, it differentiates the function two times to apply the second derivative test. In the second derivative test, keep the function f``(x) equal to zero to get inflection critical points.
At last, it puts critical points on the previous equation f(x) and you will get local maxima and minima values. Let us see an example to know the evaluation process of our extreme points calculator.
Additionally, for computations involving vector fields and gradients, consider using our gradient of a line calculator. Our calculator provides solutions for computing gradients, directional derivatives, and other vector-related operations, facilitating analysis in multivariable calculus and vector calculus.
Solved Example of Extreme Points
The extreme point can be calculated by using the extreme value calculator but it has to be done manually to know each step. So, an example is given to let you know about that.
Example:
Consider the following function and find its extrema.
$$ f(x) \;=\; 2x^3 - 3x^2 - 12x + 6 $$
Solution:
Calculate f’(x):
$$ f’(x) \;=\; 6x^2 - 6x -12 $$
Set f’(x) = 0 and solve for x:
$$ 6x^2 - 6x - 12 \;=\; 0 $$
This quadratic equation contain two critical points:
$$ x \;=\; -1 \;and\; x \;=\; 2 $$
Analyze f’’(x) = 12x - 6:
As:
$$ x = -1: f”(-1) \;=\; 12 . (-1) - 6 \;=\; -18 , is\; negative $$
So, x = -1 is a local maximum.
And,
$$ x \;=\; 2: f”(2) . 2 - 6 = 18, is\; positive $$
So, x = 2 is a local minimum.
Related: for computations involving Maclaurin series expansions and approximations, you can use our Maclaurin Series Calculator. Our calculator assists in finding the Maclaurin series representation of functions, facilitating analysis in calculus and mathematical modeling.
How to Use the Saddle Point Calculator?
Using this extreme values calculator you can easily find the extreme points of any function. It has some easy steps to calculate extrema functions that are given below,
- First, you need to enter the value of the function in the input box.
- Review the function that appears below.
- Click on the calculate button.
Looking to explore additional mathematical concepts? Try out our Puiseux Series Calculator. This calculator provides solutions for complex series functions, expanding your mathematical capabilities even further.
Result from the Relative Extrema Calculator
The extreme point calculator will give you a solution in a fraction of a second after you add input to it. With that, it gives you some extra steps to get more clarity.
- Result section will give you the solution to the extreme point function
- Possible step section provides you solution in detail
- Plot section gives a graph of extreme points in the extreme calculator
- Recalculate button will take you to a same page for the calculation.
Additionally, if you need to compute the Laurent series expansion of a function, you can utilize our laurent series online calculator. Our calculator assists in finding the Laurent series representation of complex functions, aiding in the analysis of singularities and residues in complex analysis.
Why Use the Extreme Value Calculator?
Extreme point calculator has new advanced algorithms to find complex algebraic functions extrema point solutions without any error. It has a simple design so that anyone can access it for his mathematical problem which makes our saddle point calculator best for extrema point questions.
However, this local extrema calculator is very beneficial for all. Extrema points play a key role in different fields like physics, economics, engineering, and computer science, where their values are used in decision-making and problem-solving.
Additionally, For comprehensive mathematical solutions across various domains, explore our All Calculator section, offering a wide range of mathematical tools and utilities.
Benefits of Extreme Values Calculator
To find extrema points you can use our extreme points calculator to get numerous benefits. These benefits are given as
- This extreme value calculator is easy to use because it allows you to calculate the extrema of any function within seconds.
- It is a free tool, and you don’t need any subscription fee.
- You can get the extreme point value of a particular function with a stepwise solution so that you can understand this concept properly.
- The global extrema calculator provides a more efficient solution for extreme point functions without any error.
Related: for further mathematical analyses and computations, you can go through our taylor polynomial calculator, which provides solutions for approximating functions as infinite series expansions.