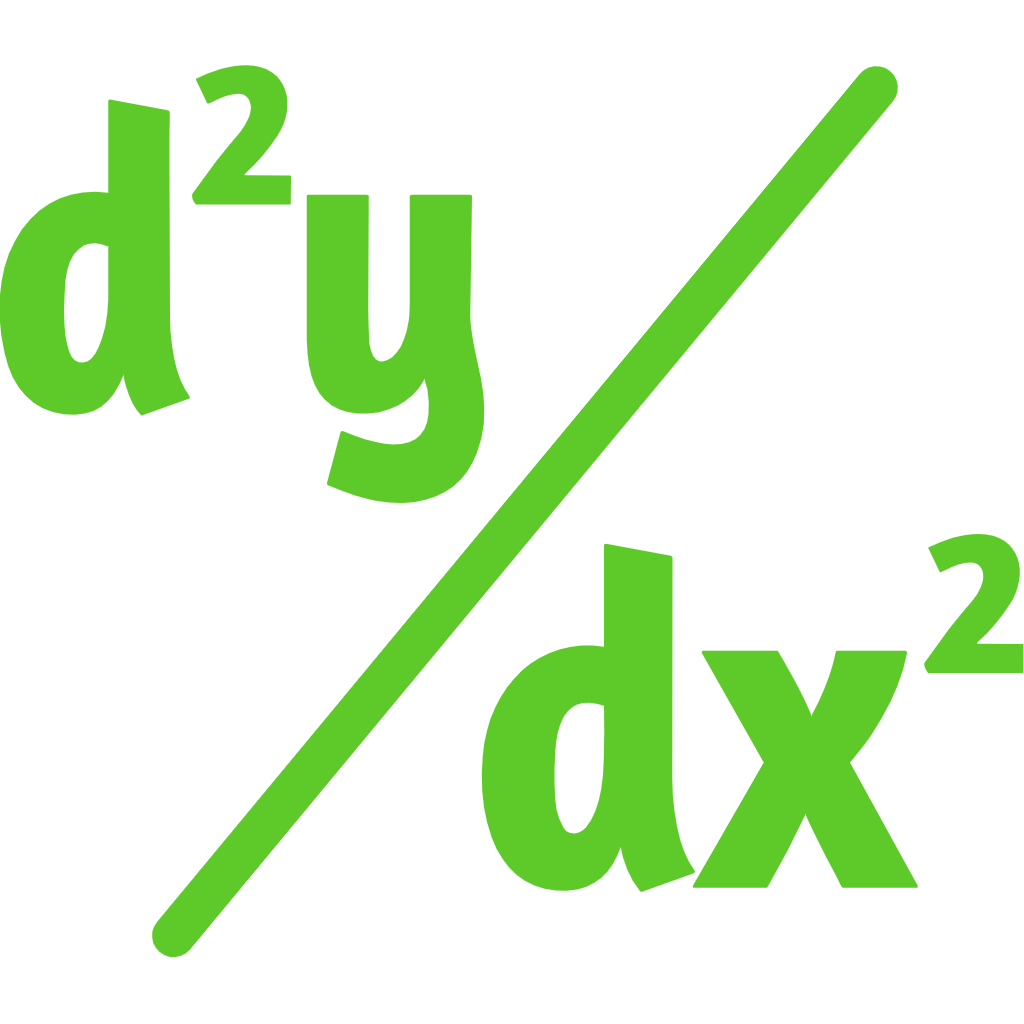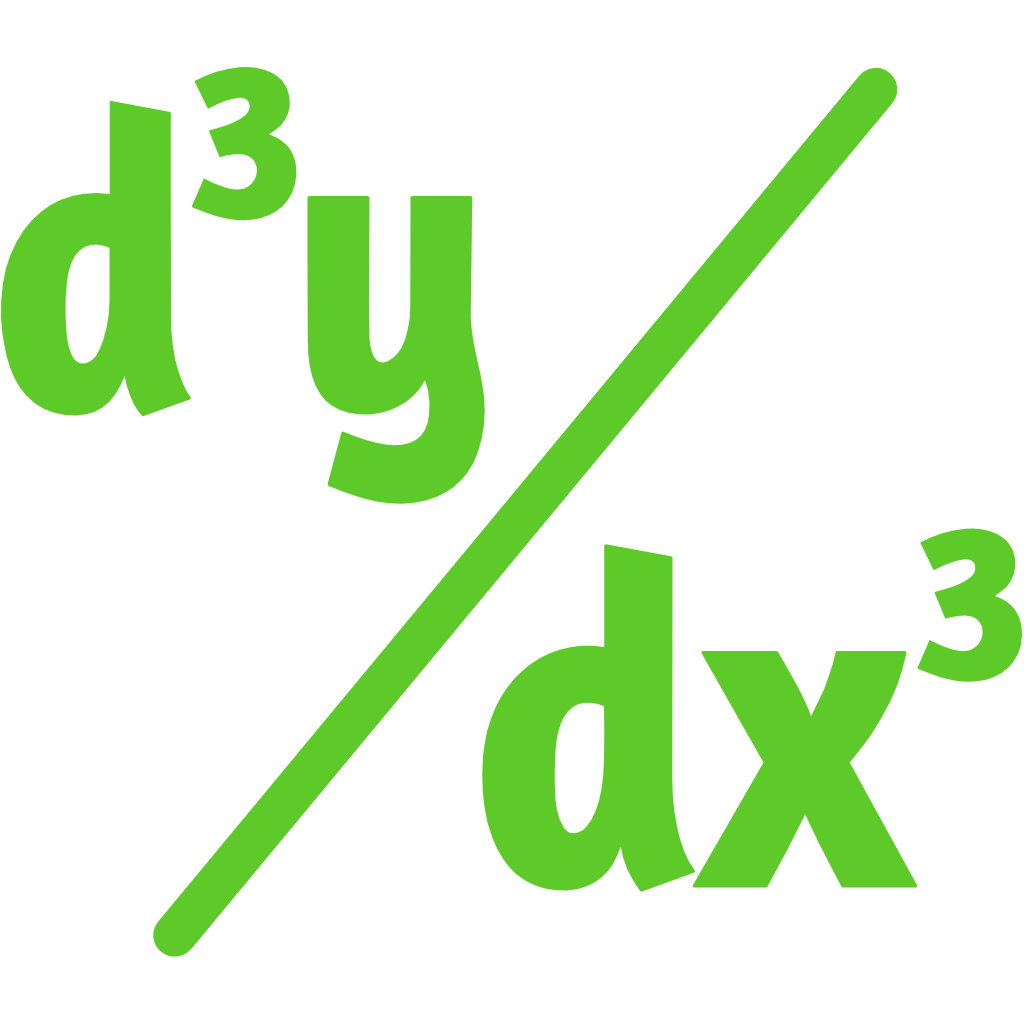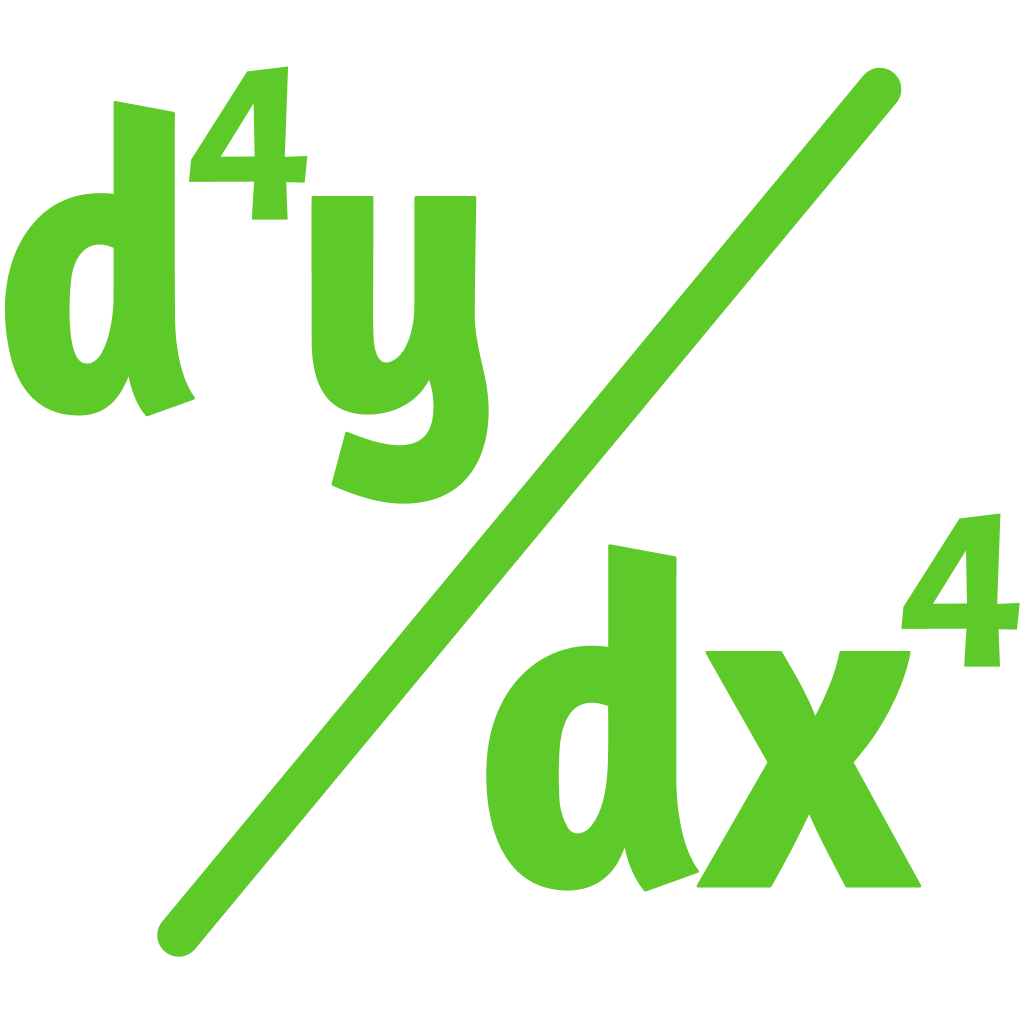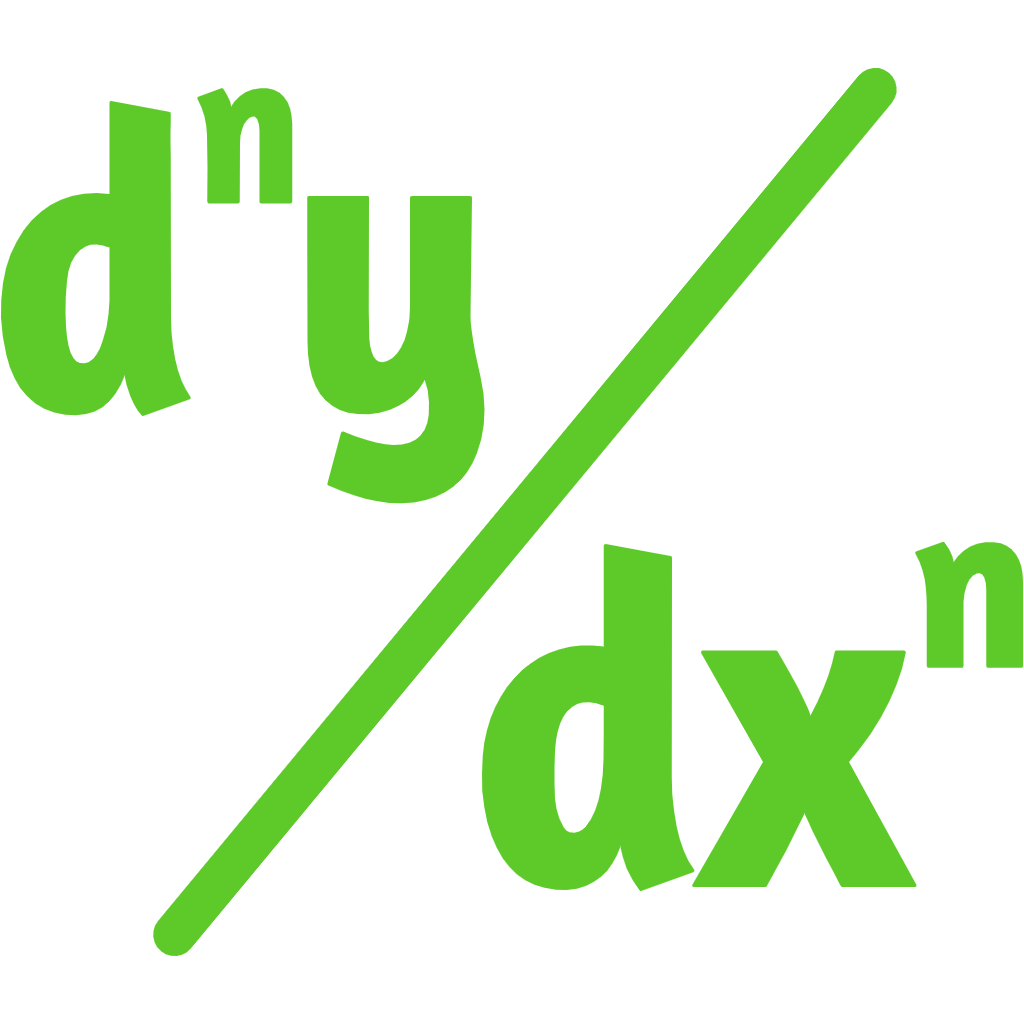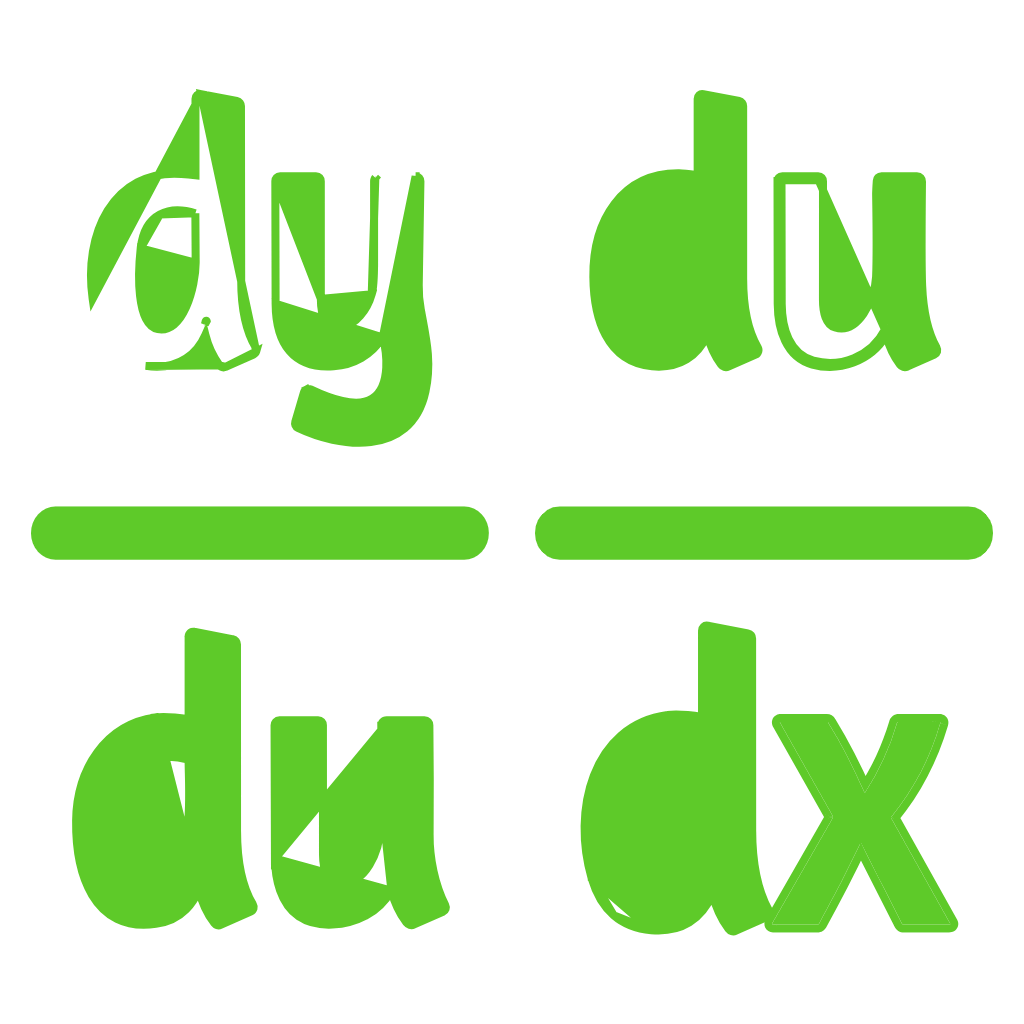Introduction to Inflection Point Calculator
Point of inflection calculator is a free online tool that is designed to find the inflection point for a given function. It helps to evaluate the inflection points from derivative concavity intervals where the curve of a function is either concave upward or concave downward.
In mathematics, an inflection point is a point on a curve where the curve changes concavity, meaning it switches from being concave upward to concave downward, or vice versa. At an inflection point, the second derivative of the function (the rate of change of the slope) changes sign.
Inflection points are crucial in various fields such as economics, physics, engineering, and biology, where understanding changes in the behavior of functions or curves is essential. Additionally, the second derivative calculator provides information about the concavity of a function, and the inflection point calculator utilizes this information to identify the points where the concavity changes, which are the inflection points. Therefore, the 2nd derivative calculator is a crucial component in the process of determining inflection points.
What is an Inflection Point?
An inflection point is a point on the curve where the concavity of a function changes its direction into a decrease or increase order due to it the concavity changes from concave down to concave up or vice versa.
Inflection points are significant because they often correspond to changes in behavior or direction in real-world phenomena described by mathematical functions.
Although inflection points are directly determined by the sign changes of the second derivative, the third derivative indirectly affects their behavior. Additionally, if you would like to calculate the third derivative of a given function. You can be use our third order derivative calculator. Our calculator also helps you to find the solution to the rate of change of acceleration problem in the run of time.
Let us see the given example to know how our points of inflection calculator calculates the inflection point of a curve with steps and how can we do it manually.
Solved Example of Inflection Point
You can find the inflection point of the curve using our inflection point calculator but it is important to understand the manual calculations as well. So we are going to give you step by step explanation of the manual calculation.
Example:
Find points of inflection of the function f(x) = x3.
Solution:
Find the first derivative:
$$ f’(x) \;=\; 3x^2 $$
Find the second derivative:
$$ f’’(x) \;=\; 6x $$
Solve x by setting the second derivative equal to zero,
$$ 6x \;=\; 0 $$
This means x = 0.
$$ For \; x \It 0, f’’(x) \;=\; 6x \It 0 \; the\; curve\;is\;concave\;down. $$
$$ For\; x \gt 0, f’’(x) \;=\; 6x \gt 0 \;the\;curve\;is\;concave\;up. $$
So it is confirmed that x = 0 is an inflection point.
Thus it is the final solution of our function with specific limits. If you would like to calculate the fourth-order derivation of a given result, you can utilize our 4th derivative calculator. This calculator helps to find the instantaneous rate of change, which increases or decreases according to its variables.
How to Use the Inflection Point Calculator?
First, find inflection points calculator online for your complex function. As you have find our calculator which has a simple interface that helps a user find inflection points function in just one click. If you follow our assistance while using this point of inflection calculator for evaluation then you will quickly find the solution.
Step 1:
Enter the point of inflection function in the input box.
Step 2:
Review the given function before calculation of the inflection point value.
Step 3:
Click the button “Calculate” to get the result of inflection points.
Additionally, if you're interested in exploring more advanced mathematical concepts, you might also find our directional derivative at a point calculator helpful. The directional derivative measures the rate at which a function changes at a given point in the direction of a specified vector.
Working Method of Our Point of Inflection Calculator:
An inflection points calculator uses a differentiation method to find the inflection point function to make a graph. When you enter a derivative equation to get the result of the inflection points in the calculator it uses a particular procedure for f(x).
First, the points of inflection calculator finds the first derivative of f(x) with respect to x. It will tell the increasing or decreasing behavior of the given function. Then it will again find the derivative f(x) and provide the concavity direction of a graph.
After derivation, put f''(x) = 0 to get the x value. If it gets the value of x then our inflection point calculator will proceed toward the next step otherwise if f(x)=0 then the given function does not exist.
After getting the value of x, a necessary condition is applied, f''(x) changes sign into positive or negative, which is f”(x)>0 then concavity is increasing and f”(x)<0, is decreasing. These inflection points show that the curve changes its concavity from up to down.
Now substitute the x-values into the original function f(x), to find their corresponding y-values. The coordinates (x, y) values are the inflection points that help to make a graph.
Related:By understanding the working method of our inflection point calculator and utilizing it alongside other advanced calculus tool like the partial differentiation calculator, you can efficiently analyze functions and explore their properties in-depth.
Results Obtained from the Points of Inflection Calculator:
You will get the result of an inflection point value with some other options for better clarity.
- The point of inflection calculator will give you results with steps.
- Possible steps section will help you know its steps.
- Plot section will show you a graph with (x,y) coordinates.
- The Recalculate button gives you a new page for more calculations.
Further, if you are interested in exploring higher-order derivatives and their applications, you can go through our multiple derivative calculator. Whether you're studying advanced calculus or conducting mathematical research, this calculator can assist you in analyzing functions with precision.
Why Choose Our Inflection Points Calculator?
An inflection point calculator is an online tool that is created to provide a smart way to understand inflection points with the help of a derivative process, which tells slope type with complete calculations.
Surely, you can calculate inflection points manually but it sometimes creates human error. Our calculator provides an accurate solution for a particular function which gives an idea about function behavior whether the tangent line rises or falls.
Additionally, For those interested in exploring more about derivatives and their applications, our solve derivative online offers a user-friendly platform for computing derivatives of various functions. Whether you're a student studying calculus or a professional needing to analyze functions quickly, this calculator can assist you in understanding the rate of change of functions with ease.
Benefits of Using an Inflection Point Calculator
Our point of inflection calculator will give you lots of benefits over other online calculators. These benefits are :
- All calculation process is done automatically as it has all built-in functions,
- The inflection points calculator reduces the complexity of getting an inflection point from a function.
- It will give a clear overview of given function solutions with steps.
- This points of inflection calculator provides a graphical representation for your visual understanding.
- This calculator can solve almost various types of functions for inflection points.
- It is a free-of-cost mathematical tool.
- Our inflection point calculator gives the results instantly, especially when working with complex functions.
Further, for an extensive collection of mathematical tools and calculators, including derivative calculators, visit our all calculators section.