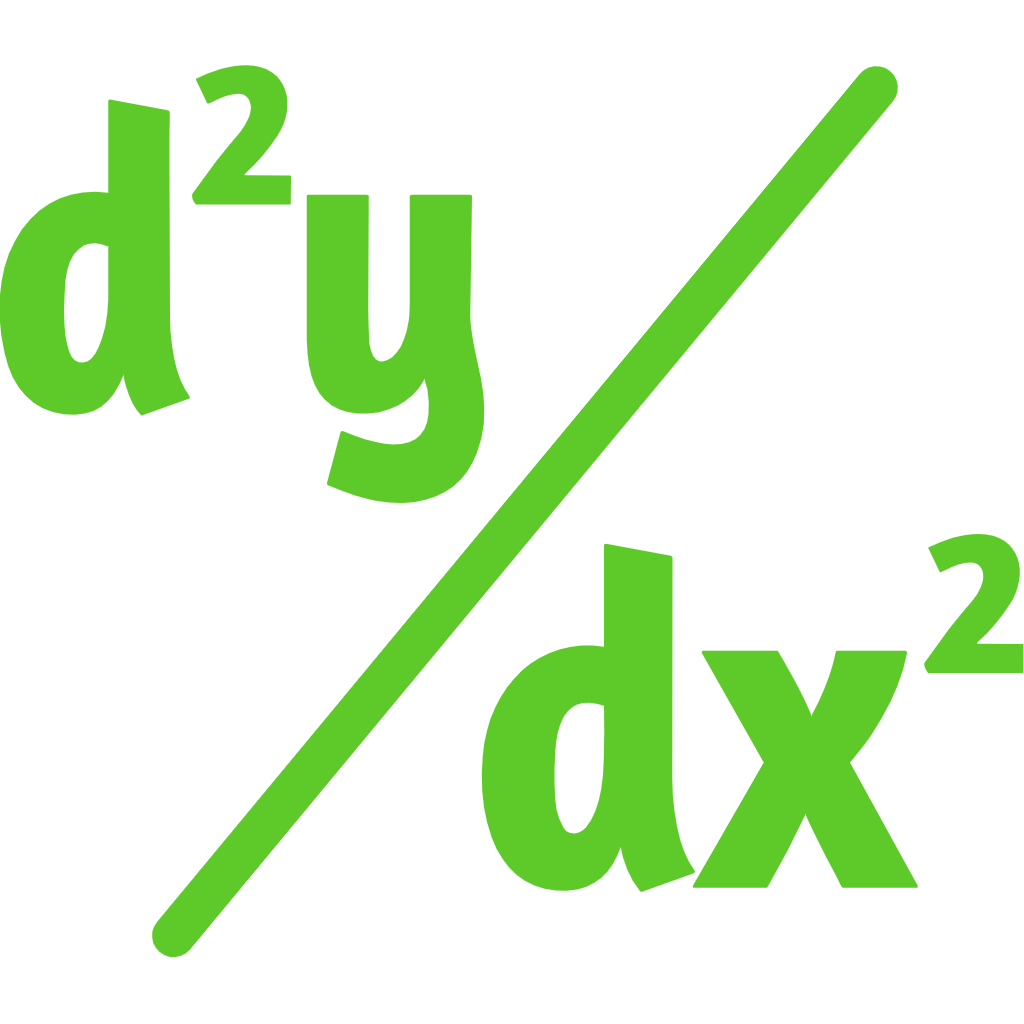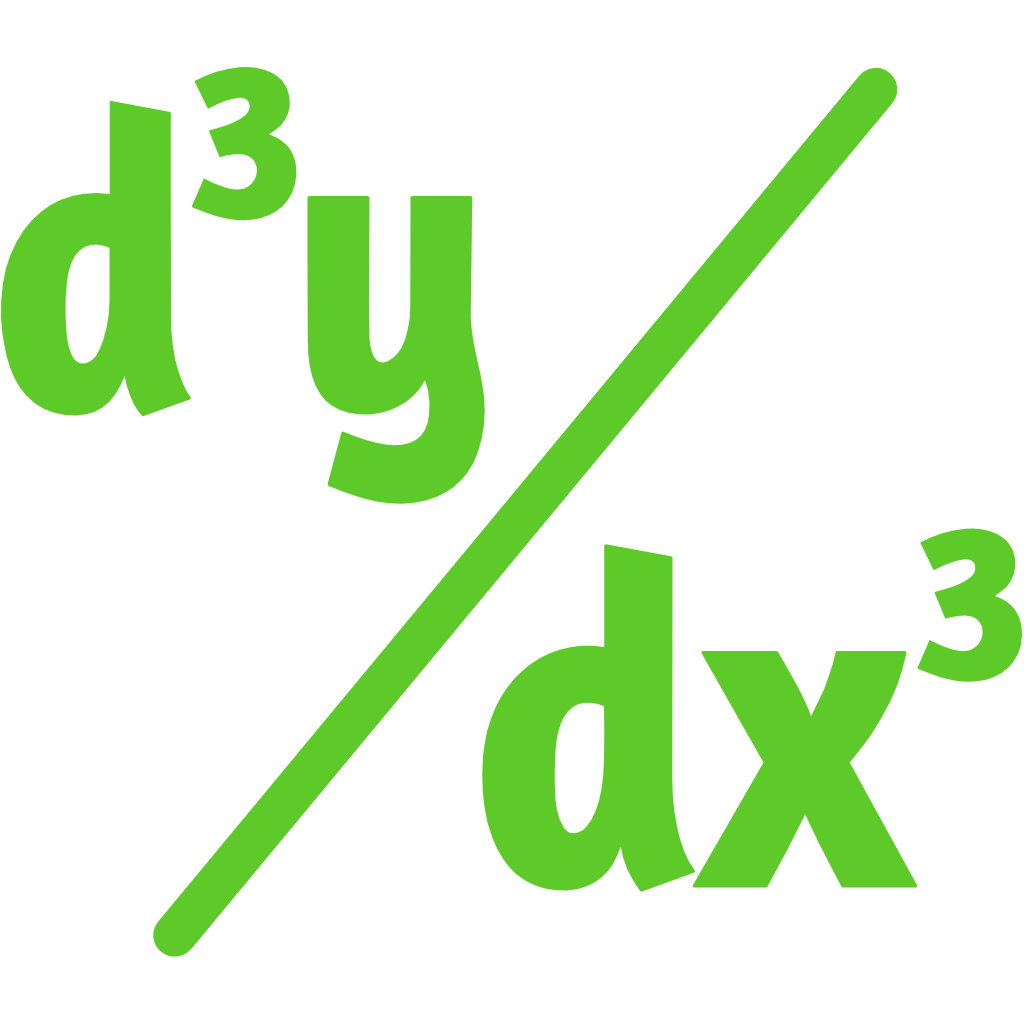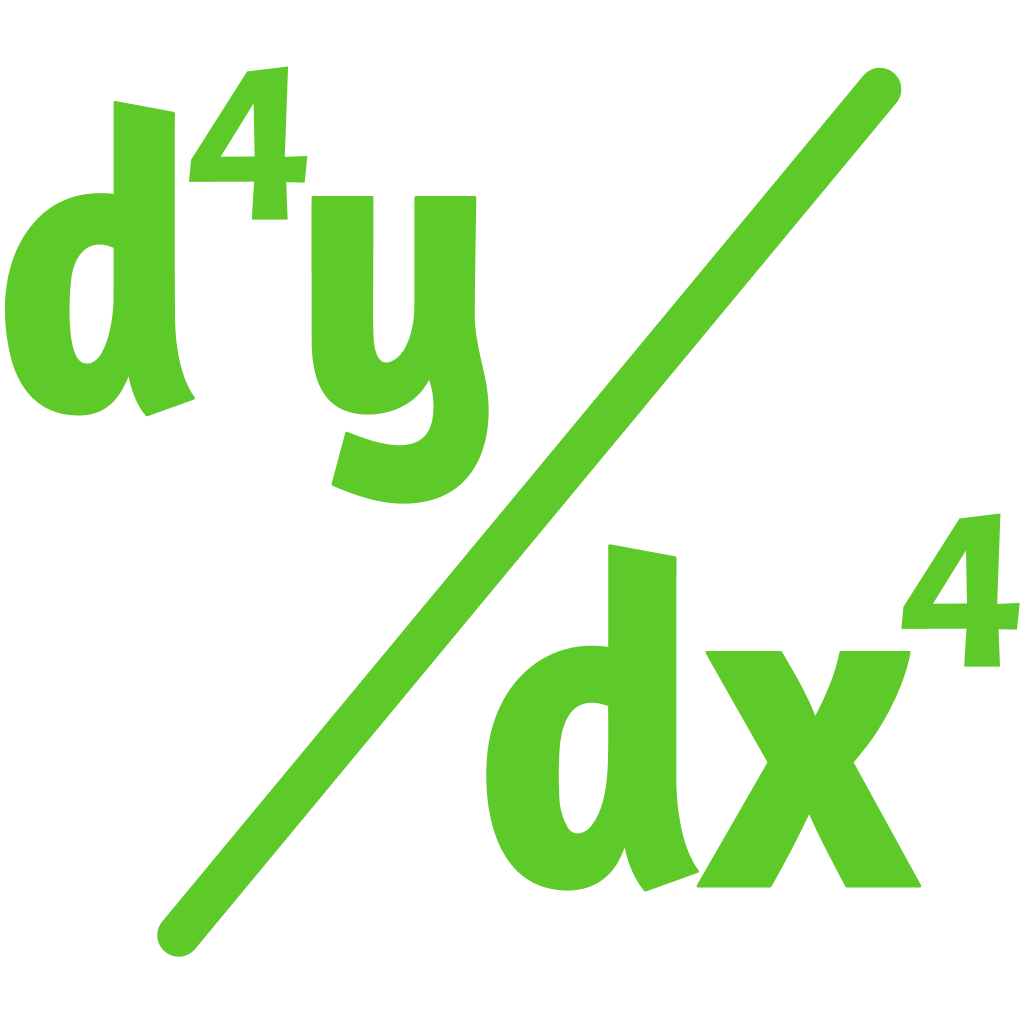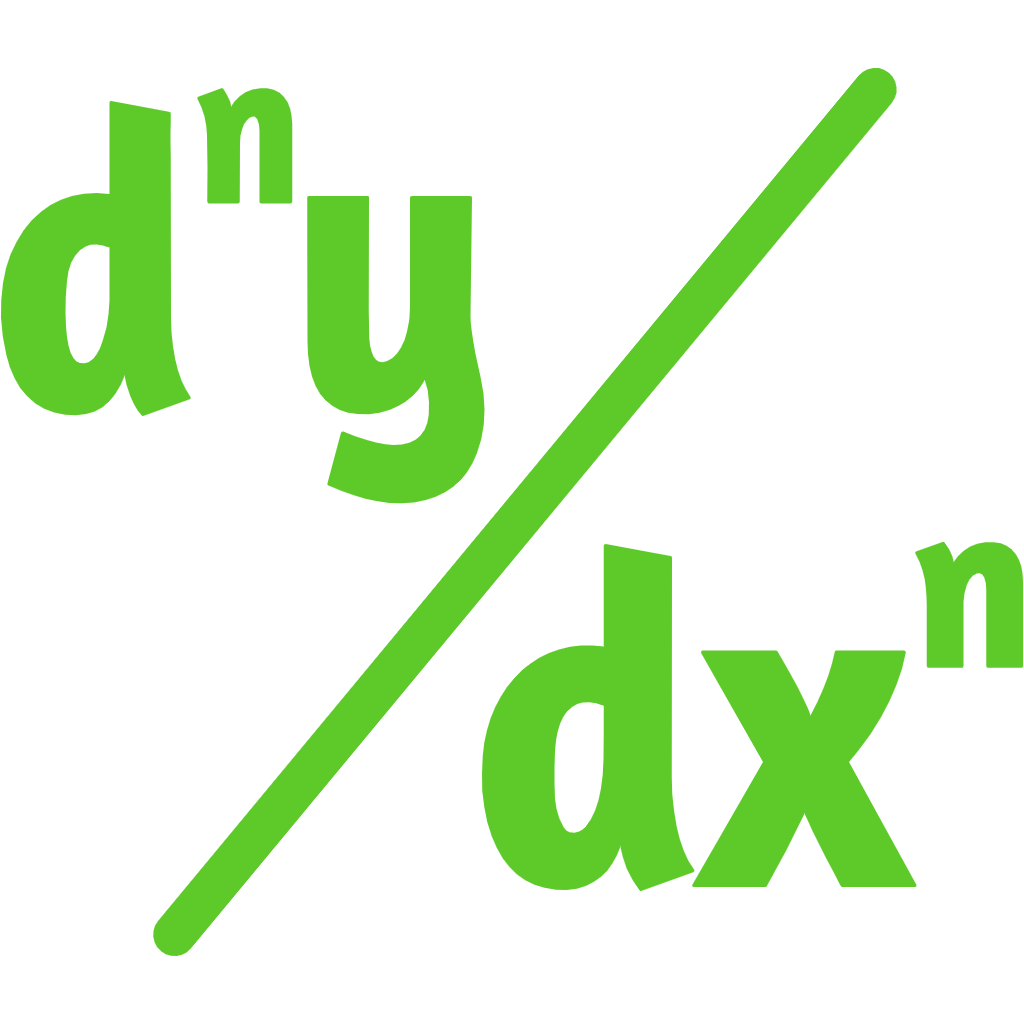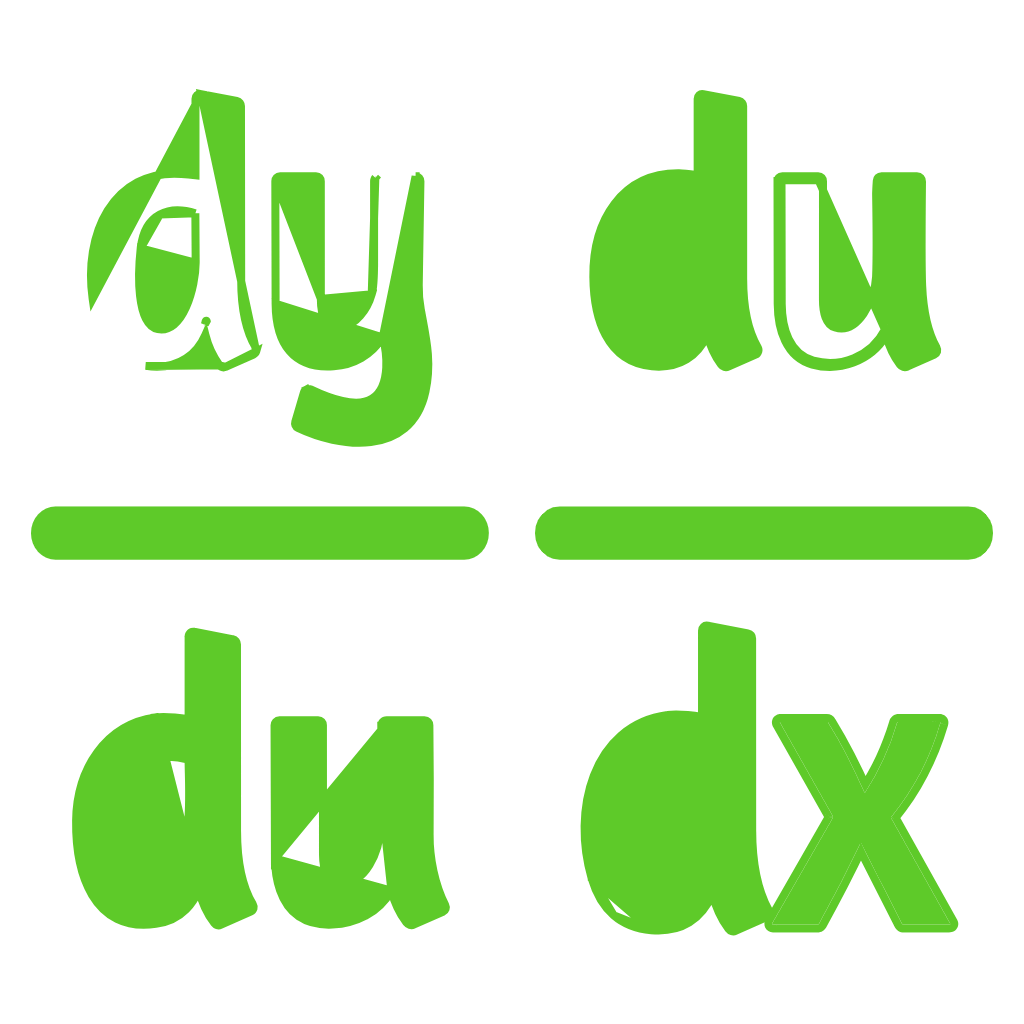Partial Derivative Calculator

Introduction to Partial Derivative Calculator with Steps

The multivariable derivative calculator is a free mathematical tool that gives the solution of the partial derivative of the function. It is used to determine the differentiation of multivariable independent functions in a run of time.

The partial derivative calculator with steps is a valuable tool for efficiently computing partial derivatives of multivariable functions while providing comprehensive guidance through the calculation process.

Our calculator is an essential resource for students, researchers, and professionals working with functions of multiple variables in fields such as physics, engineering, economics, and optimization.

Moreover, our calculator allows you to input multivariable functions with ease, supporting various mathematical expressions and functions involving multiple variables.

Further, if you would like to find second-order differentiation of a given function, you can use our second order derivative calculator. Our calculator is also used to determine whether the slope of the tangent line is increasing or decreasing on the graph.

What is a Partial Derivative?

The partial derivative is defined as a function of an independent multivariable with respect to one or more than one variable, while all other variables remain unchanged.

Partial derivatives measure the rate of change of a function with respect to one of its variables while holding other variables constant. They are essential in multivariable calculus for understanding how a function changes concerning specific variables in a multivariable context.

Our calculator also helps analyze surfaces, gradients, and optimization problems in fields such as physics, engineering, economics, and machine learning.

Additionally, if you would like to computes the third derivative of a single-variable function with respect to its independent variable, you can go through our third derivative calculator. Our calculator also helps you to find the solution to the rate of change of acceleration problem in the run of time.

Formula Used by Partial Differentiation Calculator

The partial derivative has two or more independent variables. The symbol “∂” is generally used for partial derivatives. The formula to find the partial differentiation is,

To get the partial derivation of function f(x,y) w.r.t “x”, the formula is,

$$ \frac{\partial f}{\partial y} \;=\; \frac{\partial f}{\partial u} \frac{\partial u}{\partial x} + \frac{\partial f}{\partial v} \frac{\partial v}{\partial x} $$

The partial derivation of the function w.r.t “y” can be found,

$$ \frac{\partial f}{\partial y} \;=\; \frac{\partial f}{\partial u} \frac{\partial u}{\partial y} + \frac{\partial f}{\partial v} \frac{\partial v}{\partial y} $$

Additionally, for more complex differentiation problems and to explore various derivative orders, you might use our multiple derivative calculator. The nth derivative calculator provides solutions for higher-order derivatives, allowing users to compute derivatives of any order efficiently.

Working Method of Multivariable Derivative Calculator:

Partial derivative calculator calculates partial derivatives of any order even for complex functions because all differentiation rules are installed like sum, difference, product, and quotient rules in its server.

When you give a partial differential function with multivariable in it. The multi derivative calculator analyzes the function behavior so that it recognizes which variable first it uses for derivation while treating all the other variables as constant. Then it differentiates the function with respect to the chosen variable according to the rule of differentiation.

Suppose we have a function that depends on two variables x and y given as f(x,y) in the calculator. It will first find f`(x) with respect to x and keep y as constant, again f`(y) differentiation with respect to y and x will remain constant.

In this way, the partial derivative at a point calculator gives the solution of your function. For specialized calculations involving directional derivatives and vector-valued functions, you can use our directional derivative calculator with angle. This calculator offers solutions for determining the rate of change of a function in a specific direction.

Here are some examples and step-by-step solutions to show how our partial differentiation calculator solves problems.

Example of Partial Derivative Problem

For fast results our partial derivative calculator with steps is always here but its also essential to know the manual calculation. So here is an example given to know about the manual calculations,

Example:

Solve the following:

$$ \frac{\partial^3}{\partial x^2\partial y} (e^x + e^y) $$

Solution:

First of all, find:

$$ \frac{\partial}{\partial x} (e^x + e^y) $$

The derivative of the sum/difference is the sum/difference of derivatives:

$$ \frac{\partial}{\partial x} (e^x + e^y) \;=\; \biggr( \frac{\partial}{\partial x} (e^x) + \frac{\partial}{\partial x} (e^y) \biggr) $$

The derivative of a constant is 0:

$$ \frac{\partial}{\partial x} (e^y) + \frac{\partial}{\partial x} (e^x) \;=\; (0) + \frac{\partial}{\partial x} (e^x) $$

The derivative of the exponential function is 𝜕/𝜕x (e^x) = e^x:

$$ \frac{\partial}{\partial x} (e^x) \;=\; e^x $$

Thus,

$$ \frac{\partial}{\partial x} (e^x + e^y) \;=\; e^x $$

Next is,

$$ \frac{\partial^2}{\partial x^2} (e^x + e^y) \;=\; \frac{\partial}{\partial x} \biggr( \frac{\partial}{\partial x} (e^x + e^y) \biggr) \;=\; \frac{\partial}{\partial x} (e^x) $$

The derivative of exponential is 𝜕/𝜕x (e^x) = e^x.

$$ \frac{\partial}{\partial x} (e^x) \;=\; e^x $$

Thus, 𝜕/𝜕x (e^x) = e^x

So, the next step is,

$$ \frac{\partial^3}{\partial x^2 \partial y} (e^x + e^y) \;=\; \frac{\partial}{\partial y} \biggr( \frac{\partial^2}{\partial x^2} (e^x + e^y) \biggr) \;=\; \frac{\partial}{\partial y} (e^x) $$

The derivative of a constant is 0:

$$ \frac{\partial}{\partial y} (e^x) \;=\; (0) $$

Thus, 𝜕/𝜕y (e^x) = 0

Therefore,

$$ \frac{\partial^3}{\partial x^2\partial y} (e^x + e^y) \;=\; 0 $$

So, the answer is,

$$ \frac{\partial^3}{\partial x^2 \partial y} (e^x + e^y) \;=\; 0 $$

Thus it is the final solution of our function with specific limits. Further, if you want to find fourth derivative of this partial derivative result, you can go through our fourth differentiation calculator. This calculator offers solutions for computing the fourth derivative of various functions, aiding in mathematical analysis and modeling tasks.

How do you Use a Partial Derivative Calculator?

The partial differentiation calculator has a simple interface that helps a user to easily operate it if you follow these steps while calculating your required problem.

• Enter the function with respect to x and y as input in the multivariable derivative calculator.
• Choose a variable from the given list.
• Select the differentiation order from 1 to nth order.
• Click on the "Calculate" button to get the solution of the partial derivatives.

For further exploration of derivative calculations and solutions for various functions, you may also utilize our differentiable calculator. This calculator offers a wide range of derivative computation capabilities, including single-variable and multivariable derivatives, enabling users to analyze functions with precision and efficiency.

Result Obtained from Partial Derivative at a Point Calculator

You will get the result in the run of time after giving the input in a partial derivative calculator and providing some extra options with the result.

• Result option gives you a partial derivative problem’s solution
• Possible step solution provides the solution in a step-wise process
• Plot gives a visual understanding of the given result from the graph.
• Recalculate the button to clear the previous question outcome for a new evaluation.

Related: for further analysis of functions and identification of points of inflection, you can utilize our inflection points calculator. This calculator specializes in detecting inflection points, enabling users to analyze curves and identify regions of changing concavity with ease.

Advantages of Multi Derivative Calculator:

Partial derivative at a point calculator provides multiple benefits while using it. Those benefits are,

• It gives accurate calculations while differentiating any complex function
• Our user-friendly interface gives the user the simplest procedure to use it easily.
• Our multivariable derivative calculator offers a step-by-step solution in just one click.
• It can solve all types of functions like trigonometry, and exponential, for partial derivatives

Further, for additional mathematical tools and calculators, explore our collection of all calculators, offering a wide range of solutions for various mathematical problems.

Errors while Using the Partial Derivative Calculator

Users should check these errors which are mentioned below to get accurate results from a partial differentiation calculator.

• Your expression of the function you would like to differentiate should not contain f(x) format.
• Ensure the mathematical expression which you are differentiating and there is no red error shown during evaluation.