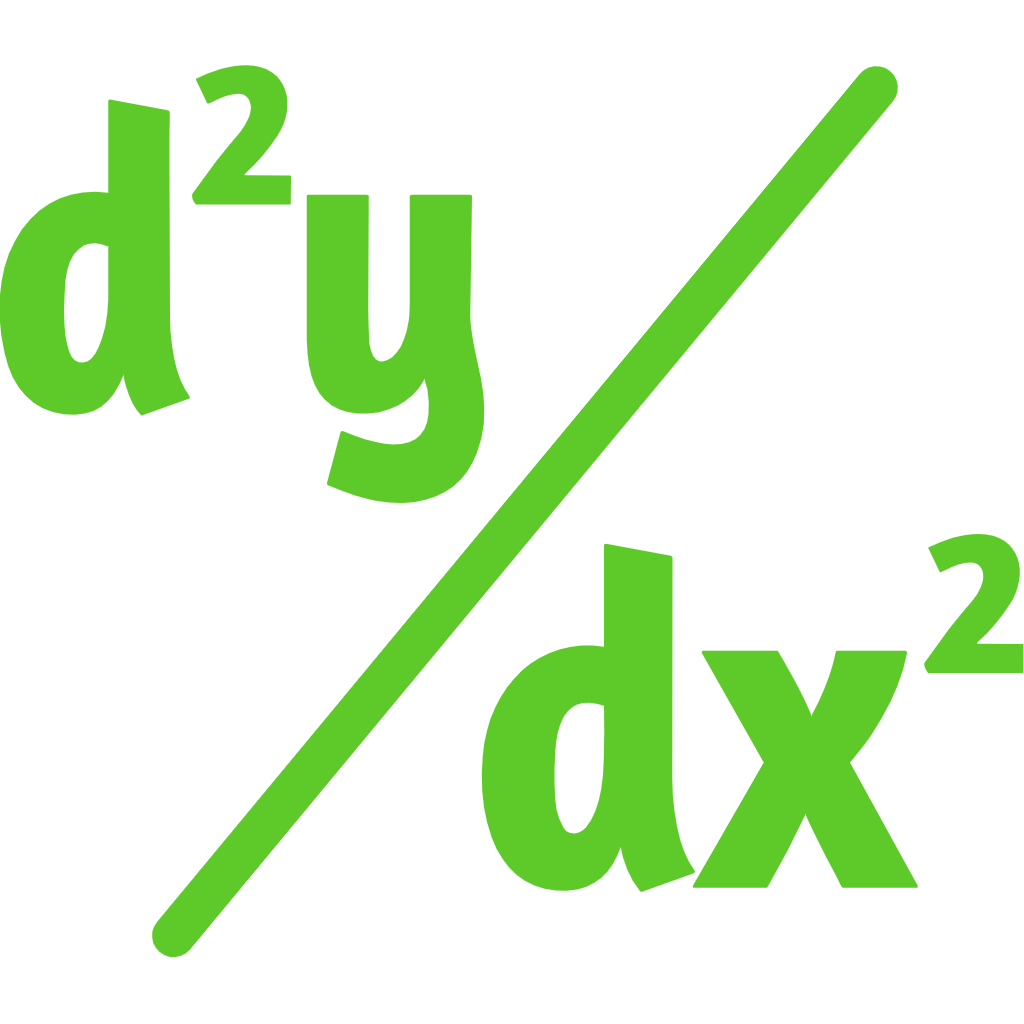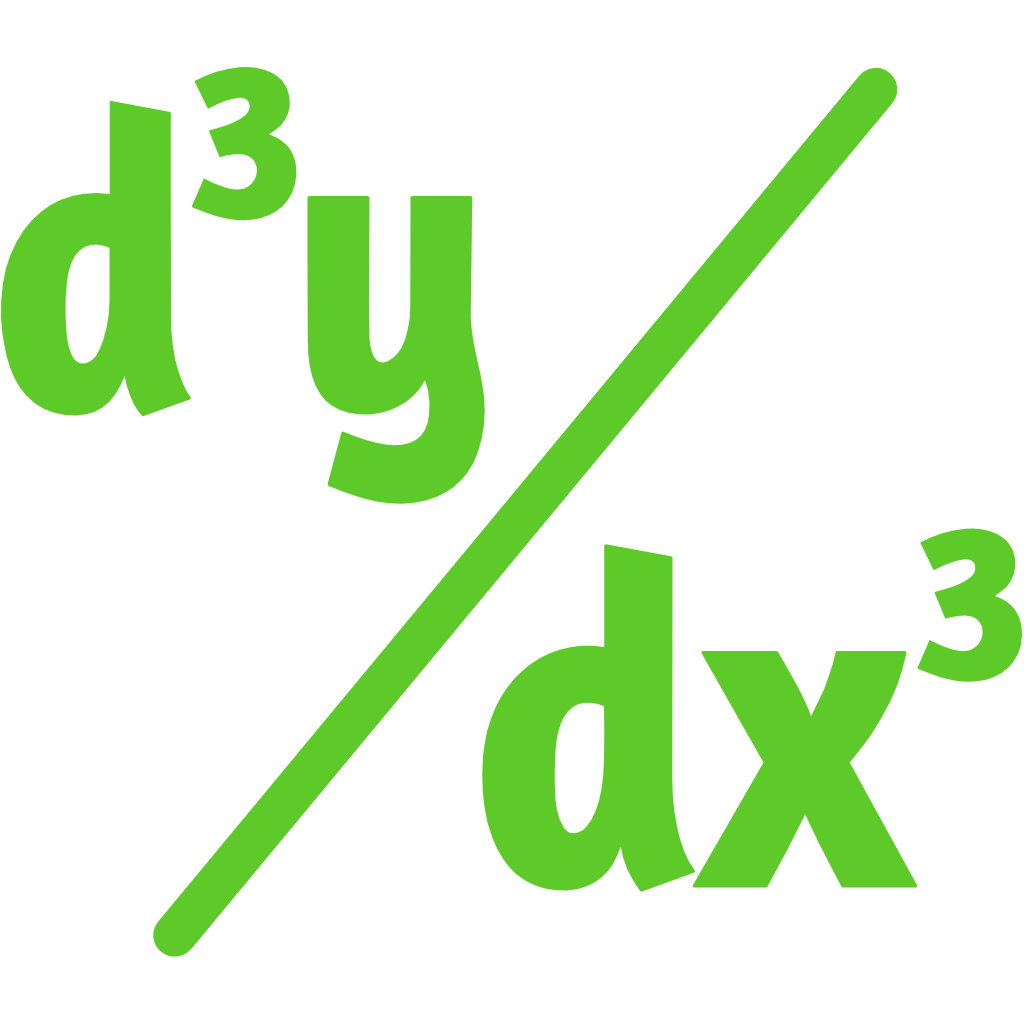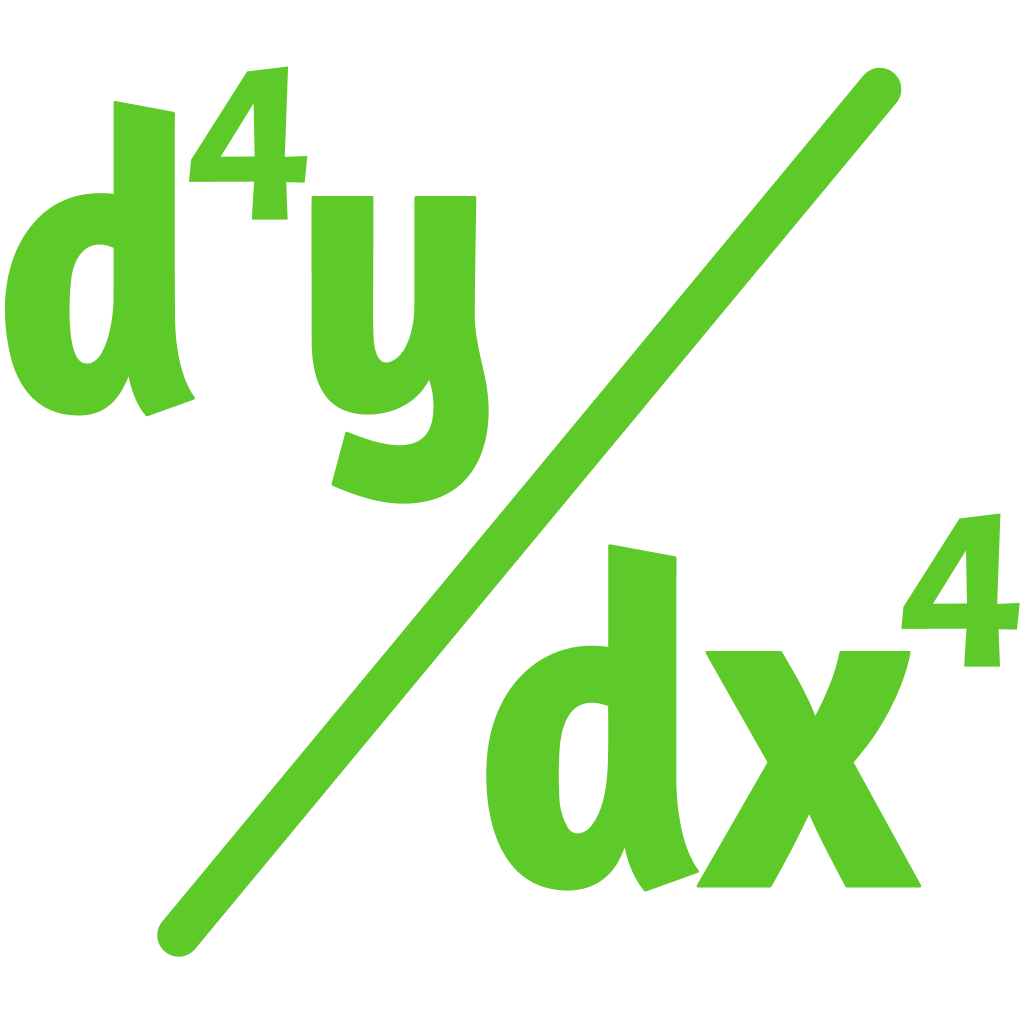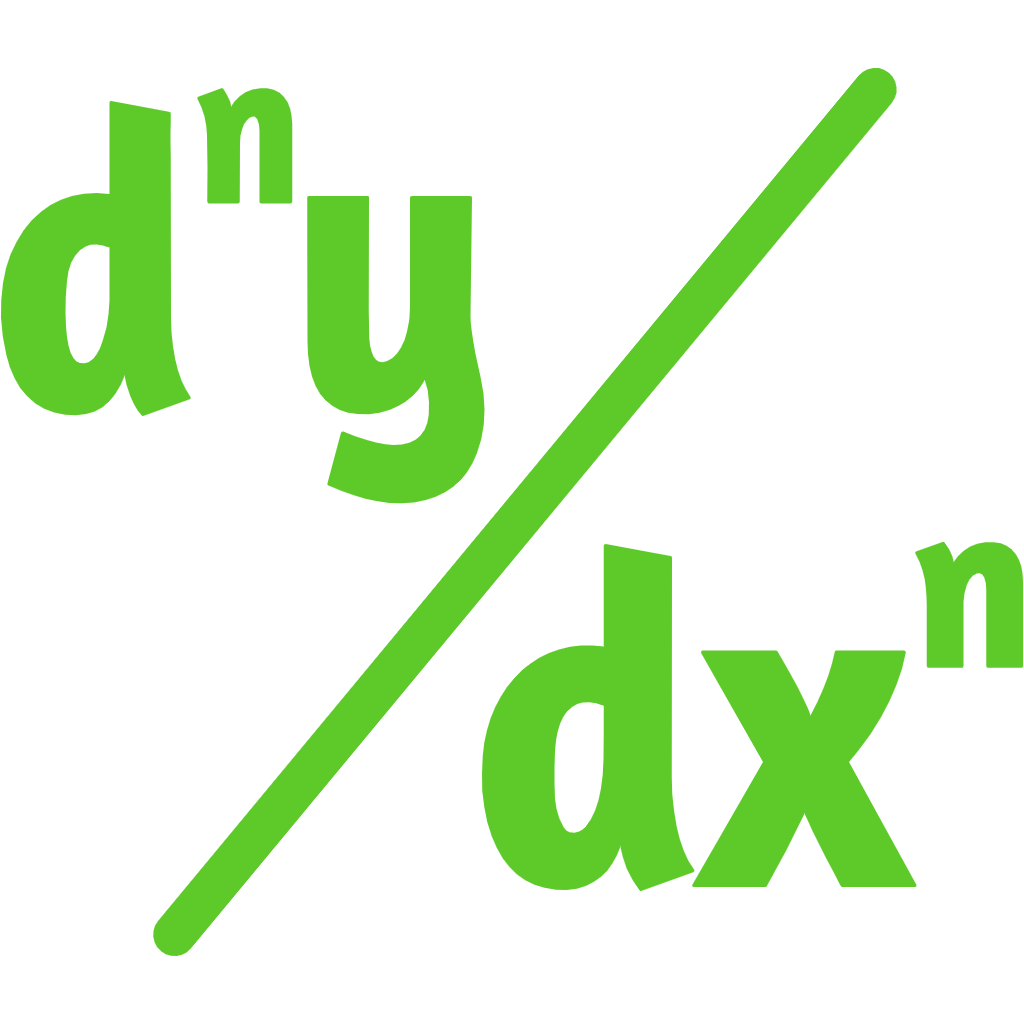Introduction to Taylor Series Calculator with Steps
Taylor polynomial calculator is a tool that is used to calculate the infinite series of a given function. It enables you to determine the complex functions of polynomials of infinite sum series in a fraction of a second.
Whether you are a student a teacher, or a researcher you need a Taylor expansion calculator to get instant solutions of infinite functions at its center point, because it is widely used in various scientific fields like engineering, physics, economics, and many more fields.
Additionally, For even more advanced mathematical computations involving singularities and complex functions, you can go through our laurent expansion calculator. This calculator is designed to compute Laurent series expansions, providing valuable insights into the behavior of complex functions in the complex plane.
Define the Taylor Series
Taylor series is a form of power series that gives the expansion of a function f(x) in the central region of a point that tells you a function is continuous or differentiated at that specific point.
The Taylor series expansion allows us to approximate a function using a polynomial, which can be easier to manipulate and evaluate than the original function. It is widely used in various fields of mathematics, physics, engineering, and other sciences for solving differential equations, numerical analysis, and modeling complex systems
Related: if you would like to evaluates the fractional exponential of a polynomial in a bounded field of nth root in a run of time, you can use our puiseux series calculator. Our calculator also provides a solution for a power series of puiseux expansion in a few seconds
Equation Behind Taylor Polynomial Calculator
The equation of the Taylor series used by the Taylor series calculator to solve the problems is given below,
$$ f(x) \;=\; \sum_{n=0}^{\infty} \frac{f^{(n)} (a)}{n!} (x - a)^n \;=\; f(a) + f’(a)(x - a) + \frac{f’’(a)}{2!} (x - a)^2 + \frac{f’’’(a)}{3!} (x - a)^3 + … + \frac{f^{(n)}(a)}{n!} (x - a)^n + … $$
For the Taylor series a sum of power series of differentiation function into n number of times that is f(x), and a is the central point f(a).
For functions approximated around the point x=0, also known as the Maclaurin series, you can be use our maclaurin series calculator. This calculator specializes in computing Taylor series expansions centered at the origin, providing efficient approximations for a wide range of functions.
Working Procedure of Taylor Expansion Calculator
Taylor series expansion calculator can solve complex functions at the given point as an infinite series, where the terms show the value of the derivative at a one-variable only. It gives you a series expansion of specific order of the polynomial function.
You just need to enter the function with its central point in the Taylor approximation calculator. It checks the nature of infinite series and then applies the differentiation rule on a given function concerning variable in n number of times as n=1,2,3.. that you specified the number for differentiation.
Now enter the center point a in f(x)=f(a) then add all n number of derivation functions to make a series. After calculation, this Taylor polynomials calculator provides you answers in the form of the sum of a series like a polynomial which helps you to understand the Taylor series concept easily.
Related:utilize the Taylor Expansion Calculator to approximate functions with polynomial series expansions, and explore further mathematical concepts with our gradient vector calculator for vector analysis.
Solved Example of Taylor Series
Taylor series can be determined by using the Taylor series calculator but it is also essential to solve it manually. So here is a manual example of the Taylor series with steps, where each step is explained in detail.
Example:
Find the Taylor series for the natural logarithm function ln(x) centered at a = 1:
Solution:
$$ f(1) \;=\; ln(1) \;=\; 0 $$
$$ f’(x) \;=\; \frac{1}{x} . f’(1) \;=\; \frac{1}{1} \;=\; 1 $$
$$ f’’(x) \;=\; -\frac{1}{x^2} . f’’(1) \;=\; -\frac{1}{1^2} \;=\; -1 $$
$$ f’’’(x) \;=\; \frac{2}{x^3} . f’’’(1) \;=\; \frac{2}{1^3} \;=\; 2 $$
Now construct the Taylor series around x = 1:
$$ ln(x) \;=\; 0 + (1)(x - 1) + \frac{-1}{2!} (x - 1)^2 + \frac{2}{3!} (x - 1)^3 + … $$
Simplify,
$$ ln(x) \;=\; (x - 1) - \frac{1}{2} (x - 1)^2 + \frac{1}{3} (x - 1)^3 + … $$
The coefficients of the series pattern allow us to write that,
$$ ln(x) \;=\; \sum_{n=1}^{\infty} \frac{(-1)^{n - 1}}{n} (x - 1)^n $$
So, this is the taylor series expansion centered at a = 1, which represents the natural logarithm involving powers of x - 1.
Additionally, for computations related to vector fields and vector calculus, including the calculation of curl, consider using our curl of vector field calculator. This calculator assists in determining the curl of a vector field, providing insights into the rotational behavior of the field.
How to Evaluate in Taylor Series Calculator
Taylor polynomial calculator is used to evaluate the differentiation method to solve a given complex series function if you follow our guidelines. These instruction are
- Enter the Taylor series function in the input box.
- Choose a derivative variable for series differentiation from the given list.
- Enter a particular point for which you evaluate the Taylor series.
- Enter the order n for approximation where n=0,1,2,3….. of series expansion
- Click on the “calculate" button for solutions of infinite series.
Further, gain insights into complex series functions with our Taylor Series Calculator, and explore further mathematical concepts with our divergence test calculator for vector analysis.
Outcome Obtained from Taylor Series Expansion Calculator
You will get a solution of infinite series after entering the input in the Taylor expansion calculator. It may include the following:
- Result section will give you the solution to the given series
- Possible Steps section provides you with solutions in steps
- Recalculate button gives you a new interface for more evaluation of the Taylor series
Why Should you Choose Taylor Approximation Calculator
Our Taylor series calculator provides accurate solutions in calculations of the Taylor Series. This tool can handle various types of series that enable you to solve multiple types of Taylor series questions. In the manual calculation, there is a high chance of error, the calculation will scrape all your efforts.
As a result, you will do the given series calculation again. It means without a calculator you cannot solve complex Taylor series questions unless you have regressed practice in Taylor series. That is why we introduce the Taylor series expansion calculator to get accurate solutions for infinite series without any trouble.
Additionally, When it comes to finding extrema (maxima and minima) of functions, you can utilize our saddle point calculator. Our calculator offers a quick and efficient way to determine critical points and classify them as local maxima, local minima, or saddle points. By utilizing this tool, you can streamline the process of identifying extrema and gain insights into the behavior of functions.
Advantages of the Taylor Polynomials Calculator
Our Taylor polynomial calculator will give you a lot of benefits for getting solutions of the infinite series without solving a long-form calculation manually. There are many advantages of the Taylor expansion calculator online which are:
- The Taylor approximation calculator provides precise calculations of the Taylor Series in no time.
- It has a simple interface which means anyone can access it.
- It provides solutions for faster calculation even for complex series.
- Our Taylor series calculator enables you to get a strong hold on the solution of series expansion for conceptual clarity.
- It does not charge anything while using it for calculation.
Explore our wide range of calculators for various mathematical, scientific, and engineering purposes. From calculus to statistics, from differential equations to numerical methods, our collection of calculators covers diverse topics to assist you in your computations. Visit All Calculators to access our complete suite of tools and enhance your problem-solving capabilities.