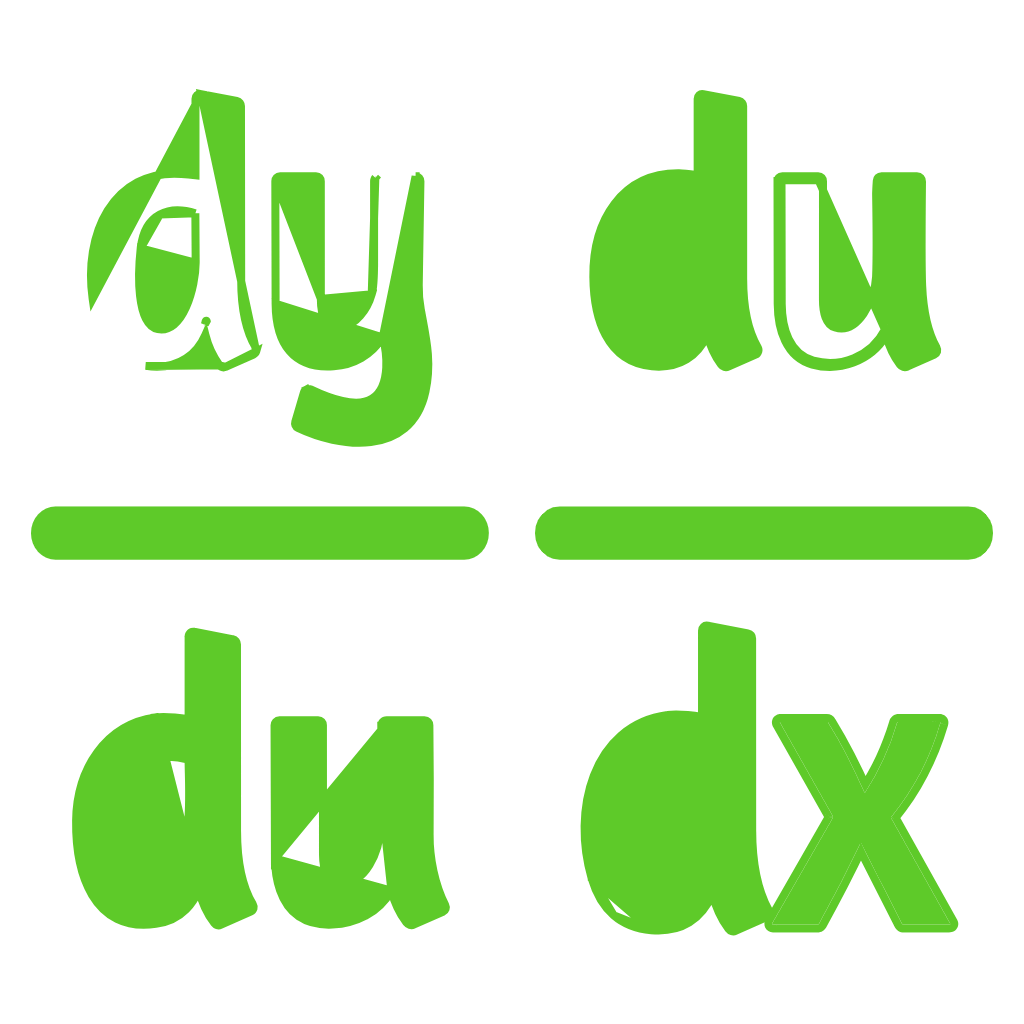Introduction to Laurent Series Calculator
Laurent series calculator is an online complex variable tool that is used to find the solution of a complex function f(z) as a series. It evaluates the analytic functions whose initial point is given and also provides the series of convergence in solution in a few seconds.
The Laurent Series Calculator empowers you to analyze and represent complex analytic functions using Laurent series expansions, offering a convenient and efficient solution for various mathematical tasks. With its accurate results, efficient processing, and user-friendly interface, the calculator is an indispensable tool for mathematical analysis and problem-solving.
Additionally, while the taylor expansion calculator is valuable for approximating smooth, well-behaved functions, the Laurent Series Calculator becomes essential for analyzing functions with singularities or discontinuities. you may choose between the calculators based on the characteristics of the function they are working with and the level of detail required in the approximation.
Definition of Laurent Series?
Laurent series is defined as the presentation of a complex function f(z) as a series of terms with non-negative powers of z. Although the Laurent series includes terms with negative powers. When the Taylor expansion is not possible to give a solution so Laurent series is used to get the solution.
The Laurent series provides a powerful mathematical tool for representing complex functions, especially those with singularities, in terms of infinite series expansions, enabling their analysis and approximation in various mathematical and scientific contexts.
While the Laurent Series Calculator is valuable for analyzing functions with singularities, the Puiseux Series Calculator becomes essential for functions with more complex algebraic singularities. You may choose between the calculators based on the specific characteristics of the function they are working with and the type of singularity it exhibits.
Notation Used by Laurent Expansion Calculator
Laurent series in complex analysis where f(z) is the analytic function that consists of two parts one is analytic function an and the other is principle part bn. The formula used by the Laurent series calculator is given,
$$ f(z) \;=\; \sum_{n=0}^{\infty} a_n (z - z_0)^n + \frac{b_1}{z-z_0} + … + \frac{b_m}{(z-z_0)^m} $$
Here,
n: the number of order of series
z: the number of singularities
z0: the initial point
an: the analytic part of the function
bn: the principal part of complex function
For computations involving series expansions centered at the origin, such as the Maclaurin series, consider using our maclaurin polynomial calculator. This calculator assists in computing Taylor series expansions centered at x=0, providing efficient approximations for a wide range of functions.
How Laurent Series Expansion Calculator Work?
The Laurent expansion calculator uses the simplest method in its working process to solve problems of the Laurent series so that you can easily understand this concept quickly. Our tool has an advanced built-in server for complex analysis that allows you to solve different problems.
When you add your input value to the Laurent series online calculator, it will first analyze the given function and initial point. Then it solves the given function first to solve both the analytic and singular parts.
Let's observe an example that is given below where the f(z) function is solved using a partial fraction to get the points of z. After partial fraction, we knew z=i and the analytic function 1/z+ibecame a geometric series. So we used a geometric series to get the values of 1/z+i.
Now, the Laurent series calculator adds the value of 1/z+i into f(z) (where partial fraction solution) to find the principle part. Then the analytic function is the singular point that shows the given function converges at z=i in no time.
Related:For computations related to vector fields and gradient analysis, you can use our gradient of a line calculator. This calculator assists in determining the gradient of a scalar field, providing insights into the rate of change and direction of maximum change of the field at a given point.
Example of Laurent Series
An example of the Laurent series is given below to let you know about the manual calculation. Although the Laurent series expansion calculator can solve difficult problems for you it is important to know the manual calculations,
Example:
Determine the Laurent series for the following around z0 = i.
$$ f(z) \;=\; \frac{z}{z^2 + 1} $$
Solution:
By using the partial fractions
$$ f(z) \;=\; \frac{1}{2} . \frac{1}{z-i} + \frac{1}{2} . \frac{1}{z+i} $$
As the 1/z+i is analytic as it has Taylor series expansion at z=i. So we will find it using geometric series,
$$ \frac{1}{z+i} \;=\; \frac{1}{2i} . \frac{1}{1+ \frac{(z-i)}{(2i}} \;=\; \frac{1}{2i} \sum_{n=0}^{\infty } \biggr( - \frac{z-i}{2i} \biggr)^n $$
So the Laurent series is,
$$ f(z) \;=\; \frac{1}{2} . \frac{1}{z-i} + \frac{1}{4i} \sum_{n=0}^{\infty } \biggr( - \frac{z-i}{2i} \biggr)^n $$
Additionally, explore the Puiseux series expansion of functions to understand their behavior near singular points, and you can be go through our curl of a vector calculator for vector field analysis in various mathematical and scientific applications.
Steps for Using the Laurent Series Calculator
The Laurent expansion calculator has a user-friendly interface that enables everyone to use this calculator to solve various types of complex analysis questions in less than a minute. For this follow some of our suggested steps so that you do not get any trouble using it. These steps are:
- Enter your analytic function in the input field.
- Add the initial value of function f(z) in the relevant field
- Click the “Calculate” button to calculate the solution of the Laurent series problems
- Click the “Recalculate” button to get a new page for more evaluation of complex variable problems.
- Review your input function before pressing the calculate button to start the process.
Related:for computations involving vector fields and divergence analysis, you can use our divergence theorem calculator. This calculator assists in determining the divergence of a vector field, providing insights into the flow and expansion of the field at a given point.
Outcome from Laurent Series Online Calculator
Laurent series calculator will get results of complex variable functions according to your given analytic function instantly after you hit the calculate button. It may be included as:
- Result box section provides the solution for your given Laurent series function
- Steps section gives you a Laurent series solution in step by step method.
Related:For computations involving the determination of extrema (maximum and minimum values) of functions, consider using our extreme points calculator. This calculator assists in identifying critical points and analyzing the behavior of functions to determine their extreme values.
Why Should you Choose our Tool?
The Laurent series expansion calculator is the best tool for solving complex analytic functions that give multiple advantages whenever you calculate different functions for the Laurent series. These advantages are:
- The Laurent expansion calculator is a trustworthy tool as it always provides you with accurate results of the Laurent series function.
- It is a speedy tool that evaluates the analytic function signature and residue a couple of times without making any manual effort for its solution.
- Our Laurent series online calculator has advanced features that allow you to solve various complex functions.
- It is a free tool so that you can use it to calculate the Laurent series problems without paying anything.
- Laurent series calculator is used for practice to solve different kinds of examples of analytic functions without any limit because it is an unlimited tool.
- It has a simple design anyone even a beginner can manage it for calculation easily.
Experience the benefits of our Laurent Series Expansion Calculator and explore a wealth of mathematical tools on our All Calculators page. Simplify your computations and elevate your mathematical prowess with ease.