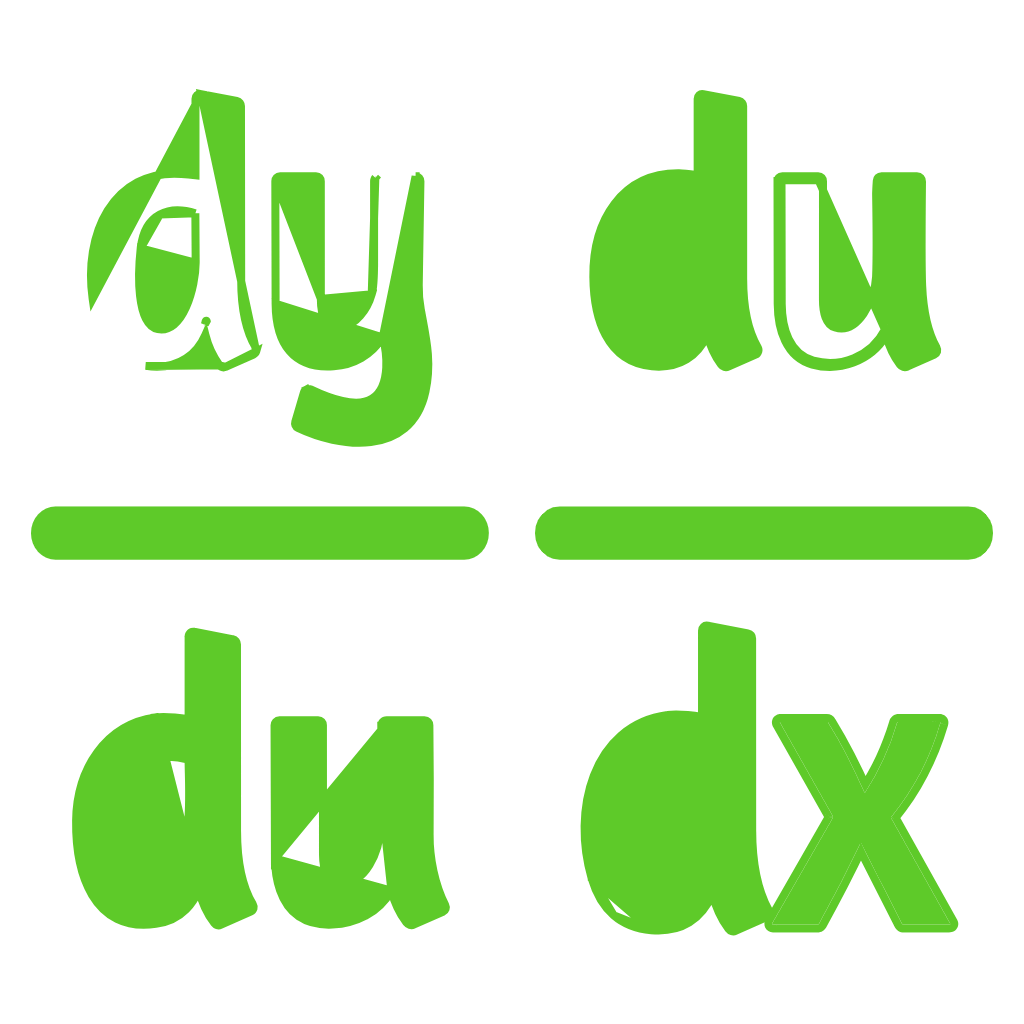Introduction to the Derivative Graph Calculator
The graph derivative calculator is an online tool that is used to plot the graph of a function with its derivative solution. It sketches the graph of a function after finding the given function derivation at every point at the interval.
The derivative plotter makes the graphing process of derivative function so simple that you better understand the differentiation concepts in a graph at every point.
This calculator is valuable for understanding the overall behavior of a function and its rate of change across its domain. It helps users identify critical points, such as maximums, minimums, and inflection points, and gain insights into the function's behavior through its derivative.
While the Derivative Graph Calculator provides a visual representation of the function and its derivative across its entire domain, our Calculator offers a specific value of the derivative at a single point. while you can use our derivative calculator at a point that provides detailed information about the function's rate of change at specific points.
This calculator is useful for obtaining precise values of derivatives at specific points, aiding in the analysis of functions and their behavior at critical locations.
What is a Derivative Graph?
The derivative graph is a graphical representation of a derivative function, to find the rate of change at every point on the interval. It refers to the relationship between the derivative and function at intervals.
The derivative graph serves as a valuable tool for understanding the behavior and characteristics of functions, particularly in calculus and mathematical analysis. It helps in interpreting the rate of change, identifying critical points, and making predictions about the behavior of functions.
A derivative graph provides valuable information about the behavior of a function and its rate of change, helping in understanding the underlying properties and relationships between different variables.
Additionally, if you would like to calculate the derivative of an inverse function, you can use our derivative of the inverse calculator. Our calculator also used to determine the complicated inverse derivation function in the simplest form.
Formula used by Graph Derivative Calculator
The formula of the derivative graph is used to plot a graph between the values of a given derivative solution along the y-axis. The formula is also used by the derivative graph calculator to find the exact solution. This formula is given below,
$$ f’ (x) \;=\; \frac{f(x + \partial x) - f(x)}{\partial y} $$
f(x) is the function of f`(x)
y is the change along y-axis
f(x+x) is the rate of change in f(x)
Related:For further exploration and to understand the concept of derivatives in more detail, you can go through our definition of derivative calculator. This calculator helps in visualizing the definition of the derivative and provides step-by-step solutions for better comprehension.
Working Method of the Derivative Plotter
The derivative graphing calculator is used to draw a graph of a given differential function that depends on the input function that you give in this tool. It has the basic derivative rules in its software to calculate the rate of change of a function.
When you enter the derivative graph function in this derivative grapher, it will first put the given function values in the limit derivative formula to find the derivation values. After getting the given function results, It uses the function values to sketch a graph at every point of the function.
Our graph derivative calculator draws a graph that shows the variation in the rate of change in the given function. After that, it compares both curve lines that give maxima or minima.
Additionally, for further exploration and to gain insight into more advanced calculus concepts, you can use our logarithmic differentiation calculator with steps. This calculator employs logarithmic differentiation techniques to differentiate complicated functions, providing step-by-step solutions for better understanding.
Now let us discuss an example of graph derivative to know how it solves the problems.
Solved Example of Derivative Graph
The plot of the graph can be found by using the derivative graph calculator but it has to be done manually. So here is an example of derivative graph.
Example:
Determine the location of all local extrema for f(x) = x3 - 3x2 - 9x - 1 by using the first derivative test. Represent it by the graph.
Solution:
To determine the critical points we have to find where f’(x) = 0. By factoring the polynomial we can conclude that the critical points must be satisfied by the polynomial.
$$ 3(x^2 - 2x - 3) \;=\; 3(x - 3)(x + 1) \;=\; 0 $$
By the above calculation, it is confirmed that the critical points are x = 3, -1. Therefore, divide the interval ( -∞,∞) into smaller ones (-∞, -1), (-1, 3) and (3, ∞).
As f’ is a continuous function, to find the sign of f’(x) for each subinterval, it chooses a point over each of the intervals (-∞, -1), (-1, 3) and (3, ∞). Find the sign of f’ for each point. For instance, let's choose x = -2, x = 0 and x = 4 only for testing the points.
f’ switches its sign from positive to negative. x increases through -1, f has a local maximum at x = -1.
f’ switches its sign from negative to positive. x increases through 3, and the local minimum is linked with f at x = 3. These results are shown on graph.
Paste Graph Here!
For further exploration and to understand the concept of slopes of curves in more detail, you can utilize our curved line slope calculator. This calculator helps in calculating the slope of a curve at a given point and provides step-by-step solutions for better comprehension.
How to Use the Derivative Graph Calculator
Our derivative plotter will give you an easy way to calculate the differentiation problems and construct the graph with the help of derivation values. You just need to follow the given steps while using the derivative grapher.
- Enter the value of the function.
- Check the derivative graph function that you enter in the input box.
- Click on the calculate button to get the answer to your given derivative function
Result Comes from Derivative Graphing Calculator
You will get solutions to your given graph derivative problems and some other options.
It may contain:
- The result along with the input function
- The plot button will give you a graphical representation
- Click on the reset button for further calculation
Related: For more advanced calculus techniques, such as calculating the second derivative of implicit functions, you can should be use our second implicit derivative calculator. This calculator provides step-by-step solutions for enhanced learning.
Advantages of Derivative Grapher
Our derivative graph calculator for graph derivative problems is beneficial when you want a graphical representation of derivation. This calculator will give many benefits that are given below:
- The graph derivative calculator has an easy-to-use interface which means everyone can manage it easily.
- You do not need to make long-form calculations by hand to plot a graph because it works automatically.
- You can also use our calculator to determine the curve line on the graph faster.
- Our derivative plotter gives an accurate graph according to the given function
- It helps you to get clarity in the derivation graph concept.
Additionally, if you would like to compute the derivative of an implicitly defined function with respect to a given variable, you can use our implicit derivative calculator. Our calculator also helps in finding derivatives when the dependent variable cannot be explicitly expressed in terms of the independent variable.
Errors while Putting Values into the Calculator
Using a derivative graphing calculator to plot a derivative graph is easy because the manual calculation commits errors. All you need is to follow the given steps to avoid mistakes when entering the function in the derivative graph calculator.
- Be careful while entering the value of the function in the calculator.
- You should use the option of the examples to know how the calculator works.
- Check your function that appears in the input field so that no error will display.
Additionally, for a comprehensive collection of calculators catering to various mathematical needs, explore our All Calculators. This centralized hub provides access to a range of calculators, including derivative graph calculators, implicit differentiation calculators, and more, offering solutions for diverse mathematical tasks.