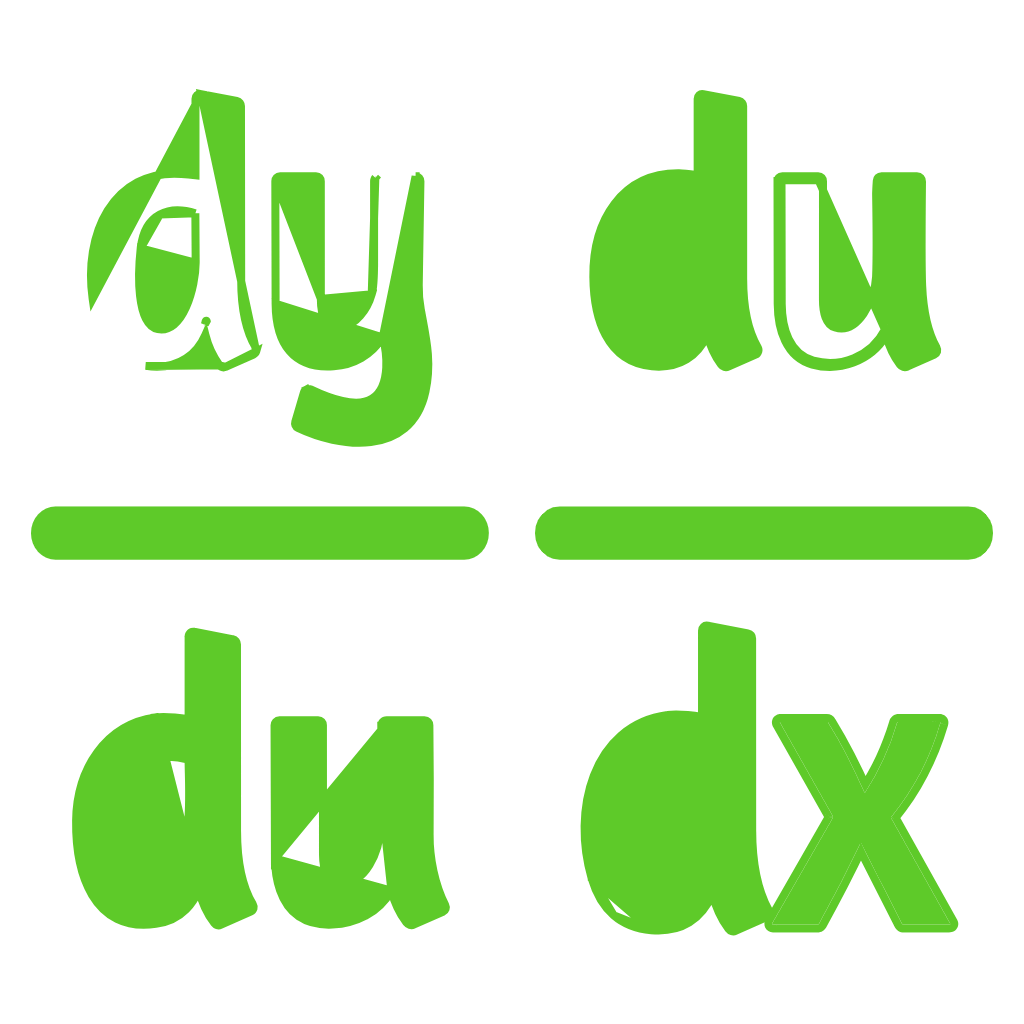Introduction to Quotient Rule Calculator
The quotient derivative calculator is a derivative tool that is used to find the quotient rule function of differentiable. It allows users to evaluate complex ratio problems using the quotient rule for derivation in the run of time.
Our Calculator is also designed to automate the process of finding the derivative of a quotient function. It simplifies the task by providing an efficient and accurate computation of the derivative using the quotient rule formula.
Additionally, by utilizing both the Quotient Rule Calculator and the product rule differentiation calculator, you can effectively navigate a wide range of differentiation scenarios, thereby enhancing their proficiency in calculus
This derivative calculator quotient rule is highly beneficial for students, teachers, and professionals who want to solve calculus problems related to quotient rules efficiently.
What is the Quotient Rule?
The quotient rule is a technique used in analytic calculus to find the derivative of a function that is present in the form of the ratio of two functions.
The quotient rule is essential for finding derivatives when direct application of basic differentiation rules (such as the power rule or exponential rule) is not feasible due to the complex structure of the function. It is commonly used in various fields of mathematics, science, and engineering to analyze and solve problems involving rates of change, optimization, and curve sketching, among others.
Further, if you are interested in exploring more about composite functions and their derivatives, our chain rule derivative calculator provides a user-friendly platform for computing derivatives of nested functions. Whether you're a student studying calculus or a professional needing to analyze functions quickly, this calculator can assist you in understanding the rate of change of such functions with ease.
Formula behind Quotient Rule Derivative Calculator
The quotient rule formula of differentiation used by the derivative quotient rule calculator involves a quotient or a fraction that is as follows.
$$ \biggr( \frac{f}{g} \biggr)’ (x_0) \;=\; \frac{f’ (x_0) g(x_0) - f(x_0) g’(x_0)}{(g(x_0))^2} $$
Where f(x) is the numerator of the derivative function
g(x) is the denominator derivation of function.
This formula provides a systematic way to find the derivative of a quotient function with respect to x. It enables the calculation of derivatives for functions expressed as the ratio of two other functions.
Related:By understanding the formula behind the quotient rule and utilizing tools like the l'hopital calculator, you can effectively tackle various calculus problems involving derivatives and limits, thereby enhancing their proficiency in calculus concepts and applications.
Working Procedure of Quotient Derivative Calculator
Derivative calculator using quotient rule enables a user to find the solution to any type of complex quotient rule problem as it has all derivative rules built-in in its software.
When you add your quotient rule problem in the form of f/g in this derivative calculator quotient rule, it will start checking the nature of the function.
After examining the function it implements the quotient rule in which at the denominator square root is applied 1/g2but at the numerator product rule of derivation is applied by adding a subtraction sign like f`*g-g`*f/g2. In this way, you can get solutions for any type of quotient rule problem of complex functions in the calculator.
By understanding the working procedure of the quotient derivative calculator, you can use our eulers method calculator, users can effectively tackle various calculus and differential equations problems, thereby enhancing their proficiency in mathematical concepts and applications.
Let us examine the quotient rule example to know how the quotient rule derivative calculator works.
Solved Example of the Quotient Rule:
The quotient rule problem can be solved with the help of our derivative using quotient rule calculator. But the problems should be solved manually sometimes. So here is an example with a detailed explanation of each step.
Example:
Find the derivative of:
$$ \sqrt{\frac{5x+7}{3x-2}} $$
Solution:
$$ \sqrt{\frac{5x+7}{3x-2}} \;=\; \frac{\sqrt{5x+7}}{\sqrt{3x-2}} $$
Applying the quotient rule,
$$ \frac{d}{dx} \biggr( \frac{\sqrt{5x+7}}{\sqrt{3x-2}} \biggr) \;=\; { \sqrt{3x-2} . \frac{d}{dx} (\sqrt{5x+7}) - \sqrt{5x+7} . \frac{d}{dx} \sqrt{3x-2} \over 3x-2 } $$
$$ { \sqrt{3x-2} . \biggr( \frac{5}{\sqrt[2]{5x+7}} \biggr) - \sqrt{5x+7} . \biggr( \frac{3}{\sqrt[2]{3x-2}} \biggr) \over 3x-2 } $$
$$ { \biggr( \frac{5 . \sqrt{3x-2}}{2. \sqrt{5x+7}} \biggr) - \biggr( \frac{3 . \sqrt{5x+7}}{2. \sqrt{3x-2}} \biggr) \over 3x-2 } $$
Taking LCM, we have
$$ { 5. (3x-2) - 3. (5x+7) \over 2(3x-2) \biggr( \sqrt{3x-2} \biggr) \biggr( sqrt{5x+7} \biggr) } $$
$$ { 15x -10 -15x -21 \over 2(3x-2)^\frac{3}{2} (5x+7)^\frac{1}{2} } $$
$$ \frac{-31}{2(3x-2)^\frac{3}{2}} (5x+7)^\frac{1}{2} $$
Related:By understanding and practicing such solved examples, you can deepen their understanding of calculus concepts like the quotient rule and effectively utilize tools like the heun's method calculator to solve differential equations.
How to Use the Derivative Quotient Rule Calculator?
The quotient derivative calculator is simply a designed tool to determine complicated derivative problems. If you follow these steps that follow as:
- Enter the numerator and denominator function at the input box
- Click the button “calculate” to get the derivative
- Review your quotient rule problem before pressing the calculator button
Answers Got from the Derivative Calculator Quotient Rule:
Quotient rule derivative calculator problem solution of your function problem in a fraction of a second after adding the input in this calculator.
- Result section will show you the solution of the quotient rule from this derivative calculator using quotient rule.
- Possible step-by-step solution of the given function.
- Plot section will show you the graphical representation of the quotient rule problem.
- Recalculate button gives you a new page for more calculations of the quotient rule problem.
Further, if you are interested in exploring numerical methods for root finding, our newton method calculator provides a user-friendly platform for approximating solutions to equations. Whether you're a student studying numerical analysis or a professional needing to solve equations quickly, this calculator can assist you in understanding and applying numerical techniques with ease.
Advantages of Derivative Calculator Using Quotient Rule
Our derivative quotient rule calculator simplifies your problems when you are using this calculator. This online mathematical tool has a simple procedure to use in some steps only
- It provides accurate calculation while differentiating any complex function
- It has a user-friendly interface so that a beginner can use it easily
- Our quotient rule derivative calculator offers quotient rule differentiating into step-by-step solution
- It saves time during the long calculation of the quotient rule problem.
Additionally, by leveraging the advantages of our derivative calculator using the quotient rule and exploring tools like the bisection calculator, you can efficiently tackle a wide range of mathematical problems, thereby enhancing their proficiency in mathematical analysis and computation.
Faults while Using the Solver:
Users should check these errors which are mentioned below while evaluating the quotient derivative problem from the derivative using quotient rule calculator.
- Your expression of the function you would like to differentiate should be placed in the correct order of numerator or denominator box.
- Select the correct independent variable for differentiation.
- Make sure the expression of the quotient rule you are differentiating is properly formatted.
For a comprehensive collection of mathematical tools and calculators, explore our all calculators. Whether you're dealing with derivatives, integrals, differential equations, or numerical methods, this collection offers a wide range of resources to assist you in your mathematical endeavors.