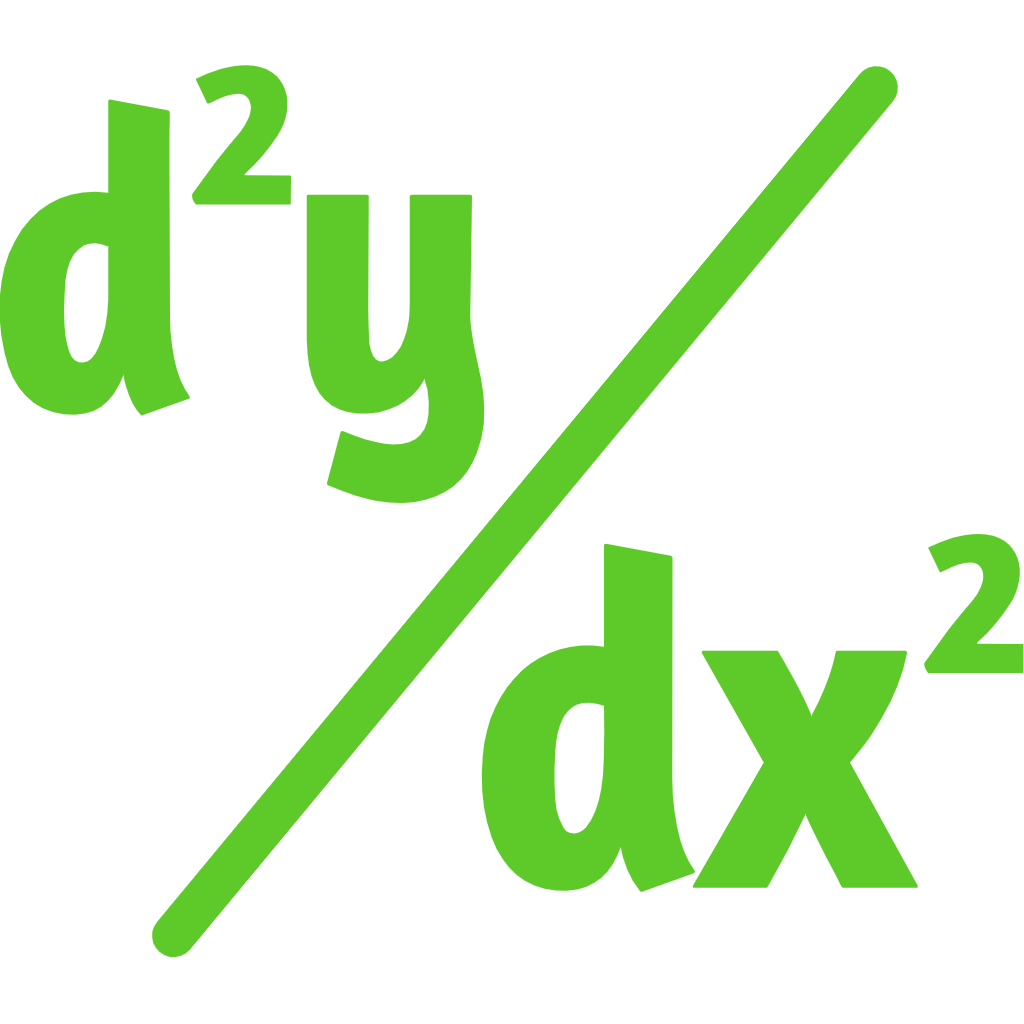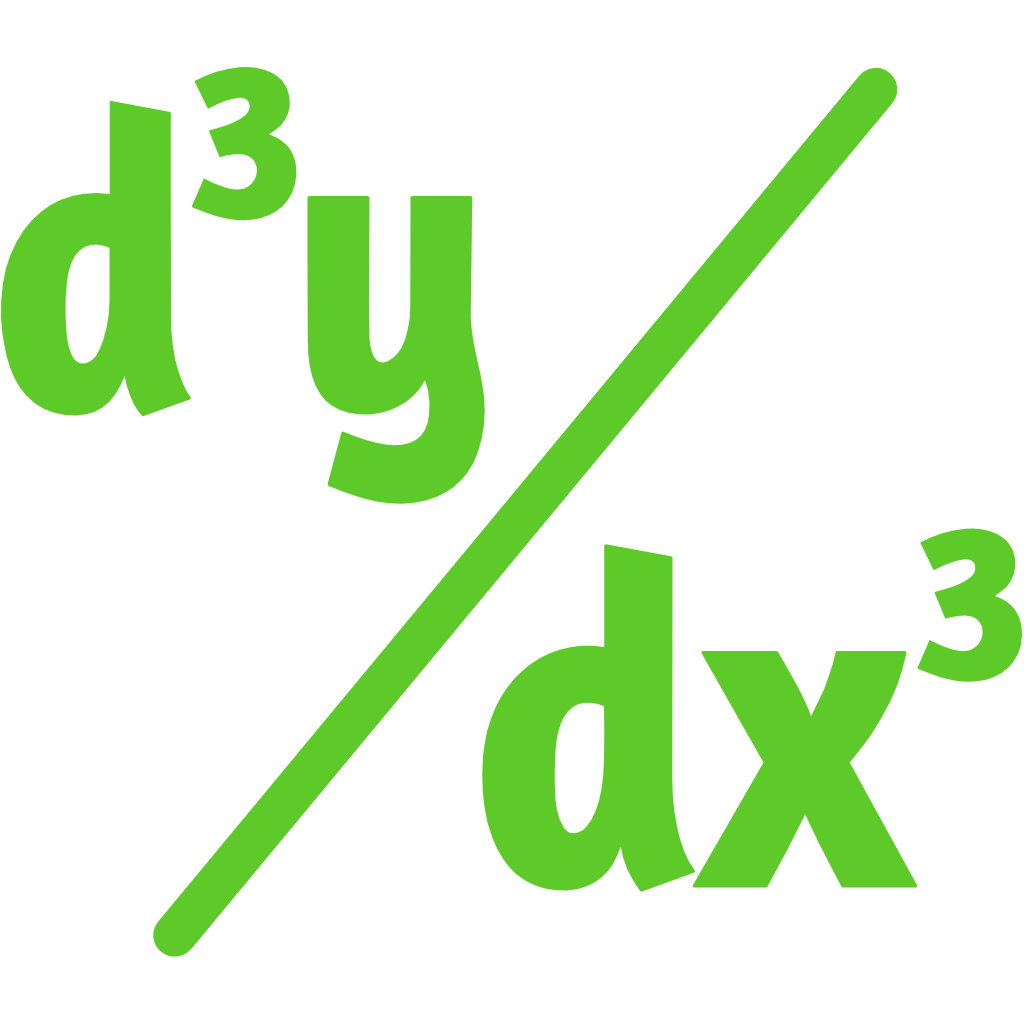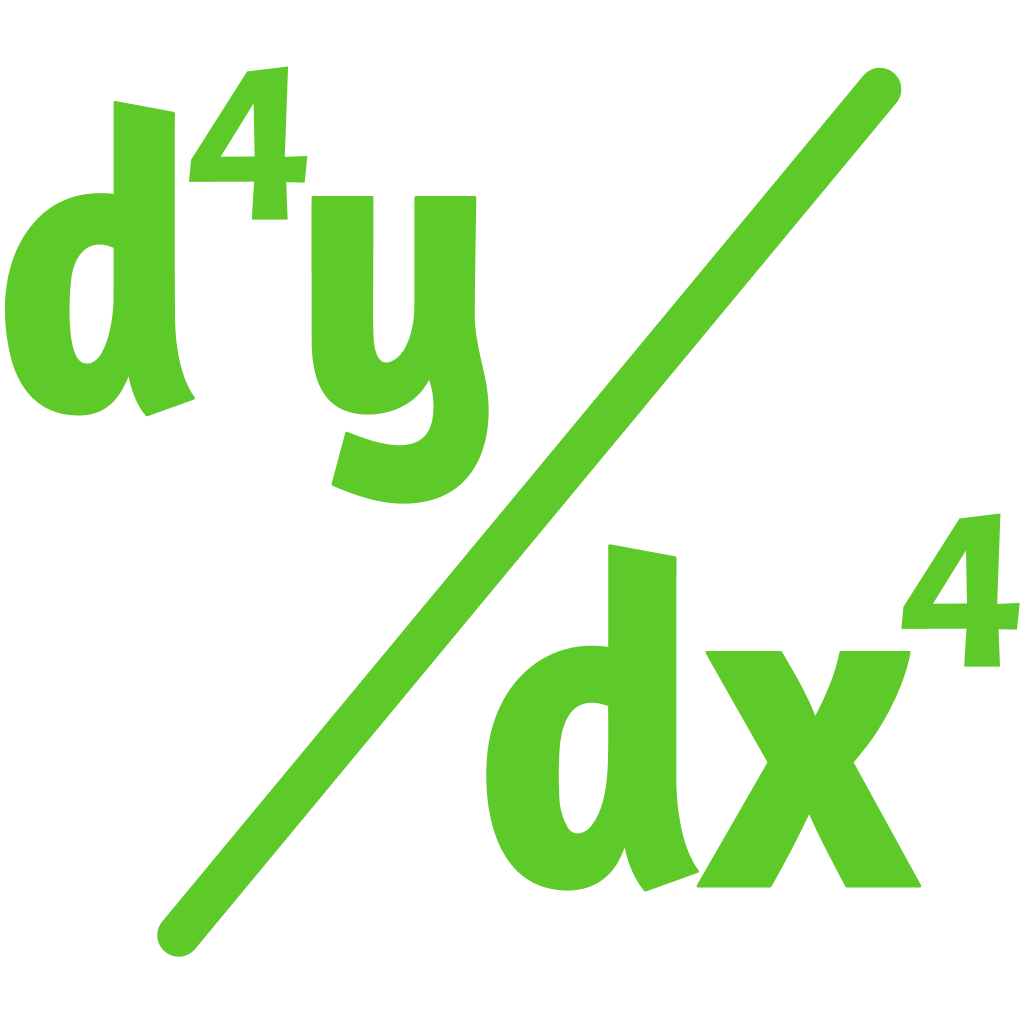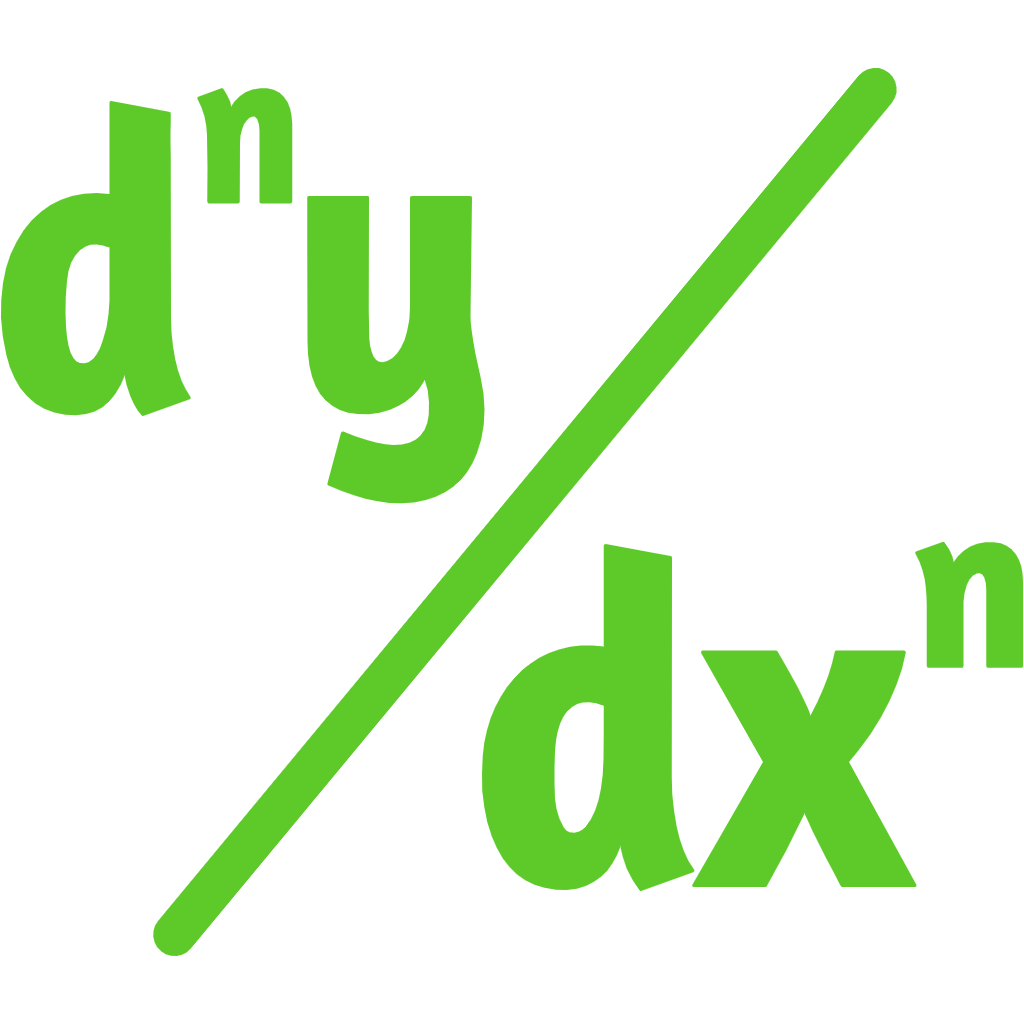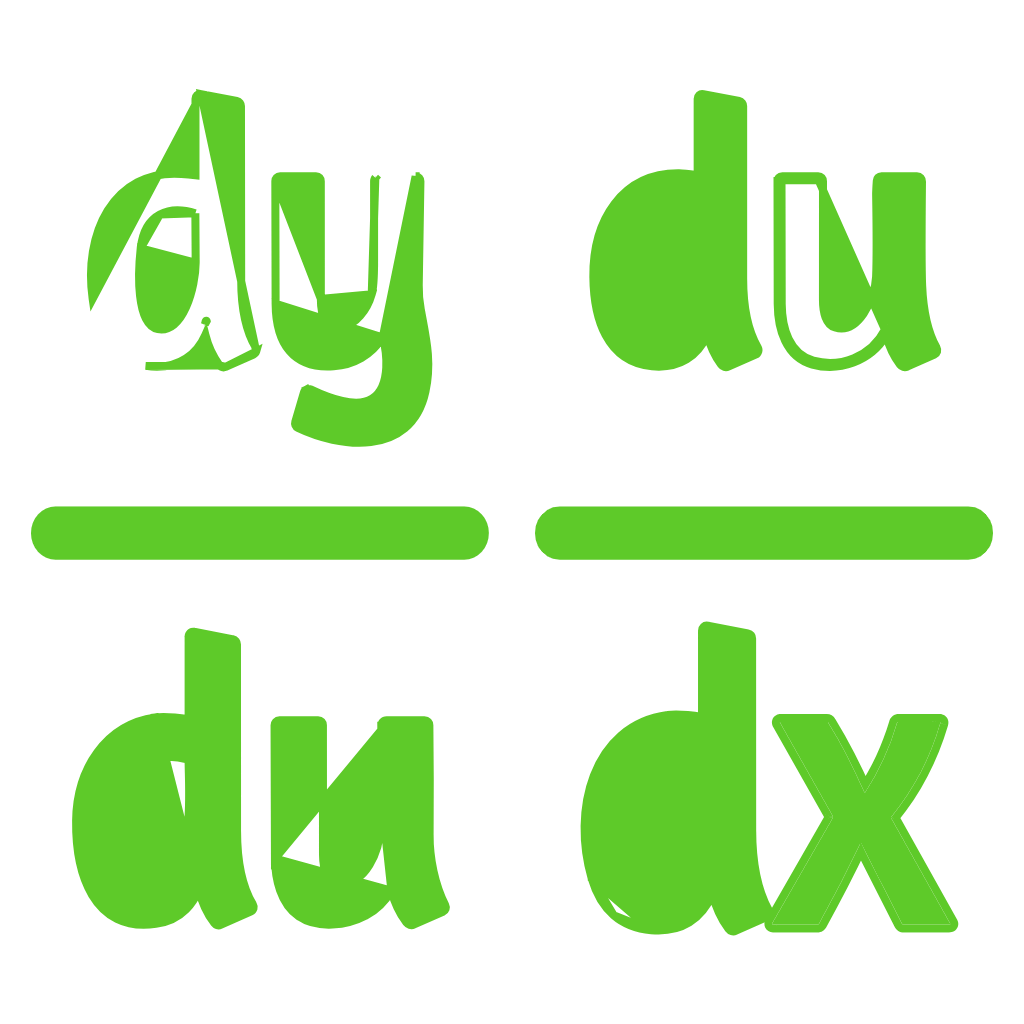Introduction to the nth Derivative Calculator
The higher order derivative calculator is a derivation tool that is used for calculating the nth derivatives of a particular function. It allows you to find the results of the instantaneous rate of change function up to the nth order in a complete step-wise procedure.
The nth Derivative Calculator is an invaluable tool for students, educators, and professionals alike, aiding in the analysis of functions and their derivatives in various mathematical contexts.
Our calculator is particularly useful in higher-level calculus, where understanding higher-order derivatives is essential in analyzing the behavior of functions.
Additionally, if you would like to find second-order differentiation of a given function, you can utilize our double derivative calculator. This calculator also used to determine whether the slope of the tangent line is increasing or decreasing on the graph.
What is a nth Derivative?
The nth derivative of a function is defined as the differentiation of the function which has n times iteration. For evaluating nth derivation order specify the function for n number of times, where n can be any real number like n=1,2,3,4,5,6……….
The nth derivative of a function is the derivative of the function taken n times successively. In calculus, functions often undergo differentiation to understand their behavior, and the nth derivative extends this concept to multiple levels of differentiation.
The nth derivative provides information about how the rate of change of the function changes as we move through successive levels of differentiation.
Further, if you would like to calculate the third derivative of a given function, you can be through our triple derivative calculator. This calculator also helps you to find the solution to the rate of change of acceleration problem in the run of time.
Notation Used by Higher Order Derivative Calculator
For the nth derivative function, differentiation notation is used whose order is in n numbers. The notation used by the nth derivative calculator is as follows,
$$ \frac{d^n}{dx^n} $$
Related:For more advanced analyses involving fourth-order derivatives and beyond, you may explore our fourth degree derivative calculator. This calculator specializes in computing fourth derivatives of functions, offering solutions for more complex differentiation scenarios.
Working Procedure of Higher Order Derivatives Calculator
Our online higher derivative calculator will calculate the derivative of any type of function as it has common rules of differentiation (product rule, quotient rule, chain rule, etc.) present in its server.
When a user gives an input function in the multiple derivative calculator, it will first analyze the function to check whether the function is differentiable n times or not. After that, it starts solving the given derivative problems as per the given iteration n=1,2,3,4,5……..
This higher derivatives calculator successively derivates the nth-order problems until the given iteration result is not obtained. This systematic approach ensures that the calculator accurately computes higher-order derivatives of functions, providing users with comprehensive solutions for their differentiation needs.
Additionally, for more comprehensive differentiation capabilities, you can go through our derivative calculator online. This versatile calculator offers solutions for various differentiation scenarios, facilitating a deeper understanding of function behavior and properties.
Let's see an example of the derivative process to know how the online nth order derivative calculator solves complex problems.
Example of nth Derivative Problem
Our nth derivative calculator will solve your problems without any hurdle and it will save time but it's also important to know each step. So here is an example with explained steps for you.
Example:
Find the following:
$$ \frac{d^n}{dx^n} \biggr[sin(x) cos (x) \biggr] $$
Solution:
$$ \frac{\partial}{\partial x} \biggr(sin(x) cos(x) \biggr) $$
Use the product rule, 𝜕/𝜕x (u,v) = 𝜕v/𝜕x u + 𝜕u/𝜕x v , where u = cos(x) and v = sin(x):
$$ \cos(x) \biggr( \frac{\partial}{\partial x} (sin(x) \biggr) + \biggr(\frac{\partial}{\partial x} (cos(x) \biggr) sin(x) $$
Using the chain rule, 𝜕/𝜕x (sin(x)) = 𝜕sin(u)/𝜕u 𝜕u/𝜕x , where u = x and 𝜕/𝜕u (sin(u)) = cos(u):
$$ \biggr( \frac{\partial}{\partial x} (cos(x) \biggr) sin(x) + cos(x) \biggr( \frac{\partial}{\partial x} (x) \biggr) cos(x) $$
Simplify the expression:
$$ \cos^2 (x) \biggr( \frac{\partial}{\partial x} (x) \biggr) + \biggr( \frac{\partial}{\partial x} (cos(x) \biggr) sin(x) $$
The derivative of x is 1:
$$ \biggr( \frac{\partial}{\partial x} (cos(x) \biggr) sin(x) + 1 cos^2 (x) $$
Using the chain rule, 𝜕/𝜕x (cos(x)) = 𝜕cos(u)/𝜕u 𝜕u/𝜕x , where u = x and 𝜕/𝜕u (cos(u)) = -sin(u):
$$ \cos^2 (x) + \biggr[ -\biggr( \frac{\partial}{\partial x} (x) \biggr) sin(x) \biggr] sin(x) $$
Simplify the expression:
$$ \cos^2 (x) - \biggr( \frac{\partial}{\partial x} (x) \biggr) sin^2 (x) $$
The derivative of x is 1:
$$ \cos^2 (x) - 1 sin^2 (x) $$
Simplify the expression:
$$ \frac{\partial^n (cos(x) sin(x))}{\partial x^n} $$
Thus it is the final solution of our function with specific limits. Further, if you want to find the partial derivative of this nth derivative results, you can use our partial derivative calculator with steps. You would apply the power rule iteratively for each derivative until you reach the nth derivative.
How to Use the nth Derivative Calculator
Higher order derivative calculator is quite simple to use because it takes simple steps for higher-order derivative problems. These steps are:
Step 1:
Enter the nth derivative function in the respective field
Step 2:
Select the variable which you want to evaluate for the nth derivative problem.
Step 3:
Choose the order of differentiation in the respective box
Step 4:
Click on “Calculate” to get the desired result of nth derivative function.
By following these steps, you can quickly and accurately compute higher-order derivatives of functions, gaining insights into their behavior and properties.
Moreover, for further exploration of derivative computations and analyses, you can also utilize our directional derivative calculator. This calculator specializes in computing directional derivatives, offering solutions for understanding function changes in specific directions.
Outcome Obtained from the Higher Derivative Calculator
You will get the result of the nth derivative after giving input in the higher order derivatives calculator. It may contain as:
- Result section gives the solution of the nth derivative.
- Steps section provide the solution to the differentiation function.
- Plot section gives the solution of the derivation function in the form of a graph.
- Recalculate button provides a new page of the nth derivative calculator for more calculations.
Related:for further analysis of functions and identification of points of inflection, you can utilize our inflection point calculator. This calculator specializes in detecting inflection points, enabling users to analyze curves and identify regions of changing concavity with ease.
Advantages of our Multiple Derivative Calculator
Our higher derivative calculator has provided many benefits to students who need research projects, assignments, etc. These advantages are:
- Higher Derivatives calculator is an online free tool that does not demand a subscription fee.
- It is easy to use anyone can use it easily to find the derivation of a function
- This nth order derivative calculator gives the derivation of any function in the nth order with accurate results.
- Our calculator provides speedy calculations of higher-order differentiation.
- Our nth derivative calculator provides results with steps.
For further exploration of mathematical tools and calculators, you may access our collection of all calculators. These calculators offer a wide range of solutions for various mathematical problems, catering to the needs of students, educators, and professionals alike.